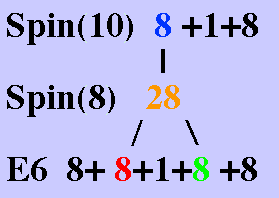

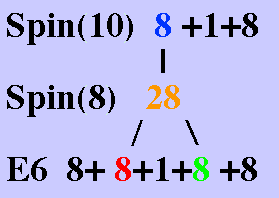
In the following diagrams Green areas are in the 130-150 GeV range, and Blue areas in the 150-200 GeV range. This histogram is of CDF events described in 1994 in FERMILAB-PUB-94/097-E: This histogram is of D0 events described in 1997 in hep-ex/9703008:
HERA H1 has observed events above the 150 GeV energy range that were not predicted by standard DIS models. HERA ZEUS has observed fewer, but similar, events. I think that the HERA events in the Blue energy range above 150 GeV are the same phenomena that have been misidentified by CDF and D0 as semileptonic Truth Quark events in the 150-200 GeV energy range. The HERA H1 events begin at 150-200 GeV:
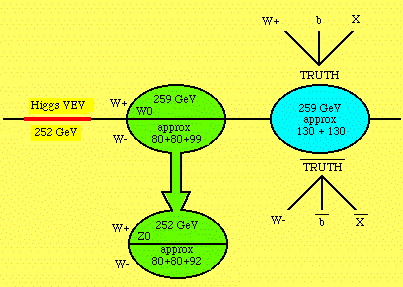
If the 150-200 GeV phenomena are not Truth Quarks or leptoquarks, then what are they? I don't know, but as more experimental data comes in, and more theoretical work is done, I hope that we will find out in due course. My personal guess is based on the observation that the D4-D5-E6 model predicts: a Higgs field VEV of about 252 GeV; a Truth Quark mass of about 130 GeV, so that a t-tbar has mass of about 260 GeV; and the sum of the masses of W+, W-, and Z0 of about 80+80+92 = 252 GeV

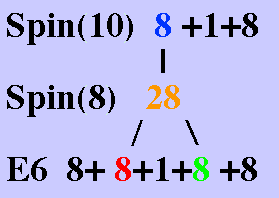
3-dim S3 = Spin(3) = SU(2) = Sp(1) Lie Algebra / \ | | \ / 3 Imaginary Quaternions i j k 1 (Associative Triangle+1) x 16 (4 signs) = 16 + 8 || 1 D4 Lattice 24 Units x2 (RL) Quaternion Products || D4 + dual D4 Lattice 48 Units
7-dim S7 EXPANDS TO S7 x G2 x S7: Spin(8) Lie Algebra / \ | | \ / 7 Imaginary Octonions i j k E I J K / \ | | \ / 7 (Associative Triangles+1) x 16 (4 signs) = 112 / \ / / \ \ 7 E8 Lattices | 224 + 16 = 240 Units \ | / x2 (RL) \ \ / / || \ / 480 Octonion Products 7 Coassociative Squares x 16 (4 signs) = 112
Due to time limits, I am going to talk mostly about some observations of Onar Aam, who made this nice octonion fractal image:
The Clifford product . for Cl(0,N) with orthonormal basis {e1, e2, e3, ... , eN} for vector a and multivector B of grade k is
Here, my notation /\ is for the exterior product, and |_ is for a "multivector interior product", corresponding to Grassmann's progressive and regressive products, which are denoted by \/ and /\ respectively in Barnabei, Brini, and Rota (J. Alg. 96 (1985) 120-160) and which are the vector space correspondents of the set theoretical union and intersection. David Finkelstein consistently uses the better notation \/ and /\ but I use /\ and |_ (worse) because it is more in line with common usage. If a and b are both vectors, then
is the sum of bivector a /\ b and scalar a |_ b CAN WE DEFINE A CROSS-PRODUCT a x b of vectors a and b such that a x b IS ALSO A VECTOR? YES, if and only if N = 1, 3, 7.
Look at the graded structure of the Clifford algebras up to N=8:
Vector Dimension
0 1 0
1 1 1 1 - COMPLEX IMAGINARIES
2 1 2 1 2
3 1 3 3 1 3 - QUATERNION IMAGINARIES
4 1 4 6 4 1 4
5 1 5 10 10 5 1 5
6 1 6 15 20 15 6 1 6
7 1 7 21 35 35 21 7 1 7 - OCTONION IMAGINARIES
8 1 8 28 56 70 56 28 8 1 8
For the 1-dim case of Cl(0,1) grade = 0 1 1 1 1 the Clifford algebra element a /\ b = 0 and a |_ b = |a| |b| DEFINE:
a x b = 0
For the 3-dim case of Cl(0,3) grade = 0 1 2 3 3 1 3 3 1 the Clifford algebra element a /\ b is in 3-dim 2-vector space and a |_ b is in 0-dim scalar space. By Hodge * duality 2-vector space is isomorphic to vector space. DEFINE:
a x b = *(a /\ b)
For the 7-dim case of Cl(0,7) grade = 0 1 2 3 4 5 6 7 7 1 7 21 35 35 21 7 1 use associative 3-vector PHI = sum of 7 associative triangles and coassociative 4-vector PSI = sum of 7 coassociative squares and a x b = *((a /\ b) /\ PSI) as the (7 - (2+4) = 1) vector. Only for N = 7 is the grade N - 2 - 1 = 4, the dimension of a 4-dim quaternionic subspace of N-space.
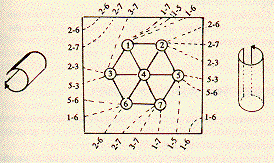
Octonion 3 Elements Associative Triangle Coassociative Square i 0001000 I J 0100000 0111000 1000111 K 0010000 I 1000000 J j 0000100 1010100 0101011 K 0010000 I 1000000 K J 0100000 1100010 0011101 k 0000010 E 0000001 i I 1000000 1001001 0110110 i 0001000 E 0000001 j J 0100000 0100101 1011010 j 0000100 E 0000001 k K 0010000 0010011 1101100 k 0000010 i 0001000 E j 0000100 0001110 1110001 k 0000010
For example, let PSI = 1000111 + 0101011 + 0011101 + 0110110 + 1011010 + 1101100 + 1110001 and calculate the imaginary octonion cross-product iK = (0001000)(0010000) = *((0001000 /\ 0010000) /\ PSI) = *(0011000)/\(1000111 + 0101011 + 0011101 + 0110110 + 1011010 + 1101100 + 1110001) = *(1011111 + 0 + 0 + 0 + 0 + 0 + 0 ) = 0100000 = J (up to +/- sign, which is determined by the cyclic ordering ijk to be -), so that iK = -J
Note that the cross product in 7 dimensions depends on the choice of the coassociative calibration 4-vector PSI, and that the rules of the cross-product vary with PSI. For imaginary octonions X and Y, the octonion product XY = X x Y - X |_ Y Any of the 480 products of octonions 1, i, j, k, E, I, J, K is based on 7 associative triangles: j / \ i---k J j J I J K / \ / \ / \ / \ / \ / \ i---K I---K I---k E---i E---j E---k THEY CORRESPOND TO THE 7 IMAGINARY OCTONIONS WHAT ABOUT THE 7 COASSOCIATIVE SQUARES? J I---j I --> / \ --> | | --> I-Onarhedron i---K k---E j J---i J --> / \ --> | | --> J-Onarhedron I---K k---E J K---i K --> / \ --> | | --> K-Onarhedron I---k j---E I J---j i --> / \ --> | | --> i-Onarhedron E---i k---K J K---k j --> / \ --> | | --> j-Onarhedron E---j i---I K I---i k --> / \ --> | | --> k-Onarhedron E---k j---J j J---I E --> / \ --> | | --> E-Onarhedron i---k K---E
HOW DO YOU BUILD ONARHEDRA? Start with an imaginary, its associative triangle, and its coassociative square. Look at the coassociative square, for example, consider i with associative triangle EIi, and the following coassociative squares associated with E, I, and i :
I-----J I-----j J-----j | | | | | | | | | | | | | | | | | | K-----E k-----E k-----K
Then notice that the opposite edges of the E-square each can be completed to an associative triangle, by putting a k at the top and bottom and a j at the left and right:
k / \ / \ I-----J / | | \ j | | j \ | | / K-----E \ / \ / k
Then identify the top and bottom k, putting it above the square, and the left and right i, putting it below the square, to form a 3-dim figure made up of the 4 triangles. Notice that the other 4 triangles, jJk, kIj, jKk, kEj, are not associative, so the 3-dim figure is not a full octahedron, just 4 alternate faces of an octahedron. Now look at the squares through the center: We started with the E-square EJIK, so it is coassociative. The other 2 are kIjE, the I-triangle, and kJjK, the i-triangle, both of which are coassociative, corresponding to imaginaries I and i. Therefore: WE HAVE 4 ASSOCIATIVE TRIANGLES AND 3 COASSOCIATIVE SQUARES. Their configuration is a HEPTAHEDRON. The 6 vertices of the heptahedron each correspond to one of 6 of the octonion imaginaries. Notice that NONE of the 3 coassociative squares EJIK, kIjE, kJjK contain the imaginary i, so that we can put the 7th imaginary i at the center of the heptahedron, and then the vertical axis of the heptahedron corresponds to the associative triangle kij = ijk .
k / \ / \ I-----J / | | \ j | i | j \ | | / K-----E \ / \ / k
WHAT ABOUT THE OTHER 3 ASSOCIATIVE TRIANGLES AND 4 COASSOCIATIVE SQUARES? With the 7th octonion imaginary at the central vertex, each of the 3 axes of the heptahedron corresponds to an associative triangle, so we get all 7 = 4+3 associative triangles. These 3 new axial associative triangles correspond to the 3 original coassociative squares, each to the coassociative square perpendicular to its axis. If we look at the 4 triangles that are opposite to the 4 associative triangles, and we combine each of them with the central vertex to form 4 tetrahedral pyramids pointing towards the 4 original associative triangles. we see that the 4 tetrahedral pyramids form 4 coassociative squares, so we get all 7 = 3+4 coassociative squares. These 4 new tetrahedral pyramid coassociative squares correspond to the 4 original associative triangles, each to the associative triangle to which it points. Now that we have all 7 imaginaries, all 7 associative triangles, and all 7 coassociative squares, WE NO LONGER HAVE A HEPTAHEDRON with 6 vertices, 4 triangular faces, and 3 square faces.
What we have now has 4 tetrahedral volumes, 20 = 7 - 3 + 16 triangular faces, 18 = 12 + 6 edges or axes, 7 = 6 + 1 vertices.
It really is 4 pyramids plus 4 triangles, where all 4 pyramids share 1 central vertex and the 6 outer vertices are each shared by 2 pyramids and 2 triangles. Its outer hull is an octahedron, and its 4 pyramids are half of the 8 pyramids of a simplicial decomposition of the octahedron. We should call this an ONARHEDRON, after ONAR, the high god in Norse mythology who created the universe, whose namesake is Onar Aam, who first worked with onarhedra. Construction of the other 6 heptahedra is similar. HERE IS A SET OF 7 ONARHEDRA, EXPLICITLY:
E / \ / \ J-----j E j J I---j / | | \ |/ I --> / \ --> | | --> i | I | i = J--I--k i---K k---E \ | | / /| K-----k K i \ / \ / E E / \ / \ K-----k E k j J---i / | | \ |/ J --> / \ --> | | --> j | J | j = K--J--i I---K k---E \ | | / /| I-----i I j \ / \ / E E / \ / \ I-----i E i J K---i / | | \ |/ K --> / \ --> | | --> k | K | k = I--K--j I---k j---E \ | | / /| J-----j J k \ / \ / E k / \ / \ I-----J k J I J---j / | | \ |/ i --> / \ --> | | --> j | i | j = I--i--E E---i K---k \ | | / /| K-----E K j \ / \ / k k / \ / \ J-----I k I J K---k / | | \ |/ j --> / \ --> | | --> i | j | i = J--j--E E---j I---i \ | | / /| K-----E K i \ / \ / k i / \ / \ K-----J i J K I---i / | | \ |/ k --> / \ --> | | --> j | k | j = K--k--E E---k J---j \ | | / /| I-----E I j \ / \ / i I / \ / \ J-----k I k j I---J / | | \ |/ E --> / \ --> | | --> i | E | i = J--E--j i---k K---E \ | | / /| K-----j K i \ / \ / I
To make an ONARHEDRON, start with a HEPTAHEDRON. A heptahedron is a polyhedron of 7 faces, 4 triangles and 3 squares (singular because the square faces intersect each other), 12 edges, and 6 vertices. If the 6 vertices correspond to 6 of the 7 octonion imaginaries, then the triple intersection in the center corresponds to the 7th. Its Euler number is 2, and, like the Klein bottle, it is 1-sided. It can be continuously deformed, not to a sphere, but to the 4th-order Roman surface of Steiner whose equation is y^2 z^2 + z^2 x^2 + x^2 y^2 + xyz = 0 In 3-dimensional space, a Roman Steiner surface is singular, but it can be embedded in 4-dimensional space without singularity, as can a Klein bottle. (See, for example, Anschauliche Geometrie by Hlbert and Cohn-Vossen, English translation is Geometry and the Imagination, Chelsea 1952, chapter VI, section 46.) A Roman Steiner surface looks like this, showing corresponding positions of a configuration of octonion imaginaries: The symmetries of the Heptahedron, and of the Roman Steiner Surface, are related to the 480 octonion products. The Heptahedron non-orientability may be related to the Escher Mobius Triangles of Squares of Onar Aam, who has, as far as I know, discovered these geometrical relations. To get an ONARHEDRON from a HEPTAHEDRON: If you include the 3 singular axes of the heptahedron and its central singular point, then, as Onar Aam noticed, not only does the central point make a 7th imaginary, but also the 3 axis-diagonals make up 3 more associative triangles, for a total of 7, and the 4 octahedral faces that are missing in the heptahedron (each with 3 points) together with the central point (all 4 forming a tetrahedron) make up 4 more coassociative squares, (including the coassociative square corresponding to the element at the central point) also for a total of 7, and that makes an ONARHEDRON, which includes the heptahedron and represents: 7 imaginaries, 7 associative triangles, and 7 coassociative squares.
Young was a mathematics student of Veblen at Princeton. For a time after Young was graduated, he concentrated on such things as inventing the Bell helicopter. After that, he devoted more attention to philosophy and mathematics. After Veblen's death, Young read a paper by Veblen and John Young (no known relation to Arthur Young) in which they stated that Projective Geometry has 7 Axioms:
Young also describes the 7 Associative Triangles, calling them Triads or Committees, and the 7 Coassociative Squares, calling them Tetrads.
Young also describes the Hexaverton, an intermediate form between the Heptahedron and the Heptaverton, as having 6 vertices and 15 edges, being 6 vertrices all connected to each other. The 6 vertices are the 6 vertices of an Octahedron, without the central vertex. The 15 edges are the 12 edges of an Octahdron plus the 3 full diagonals of an Octahedron. Young shows that the Hexaverton is topologically a Mobius Strip.
Young also describes the Pentaverton as 5 vertices all connected to each other by 10 edges, forming a 4-dimensional simplex; and the Tetraverton as 4 vertices all connected to each other by 6 edges, forming a 3-dimensional tetrahedron.
Robert de Marrais in his paper math.RA/0207003 says:
"... The following diagram of a box-kite doesn't have signed edges, and has peculiar symbols on its vertices, and its "sails" lack coloring; it is otherwise, however, indistinguishable from that provided above. This was hardly the intent of its creator, though: for it is an aid in visualizing a key proof concerning the symmetries of the curvature tensor in John Milnor's classic lecture notes on Morse Theory. ... Using the notation of affine connections, Milnor states in a lemma that a Riemannian manifold's curvature tensor satisfies four relations:(1) it is skew-symmetric (R(X,Y)Z + R(Y,X)Z = 0);
(2) cyclically ordered such triples of vector fields sum to zero (R(X,Y)Z + R(Y,Z)X + R(Z,X)Y = 0);
(3) bracket relations are skew-symmetric (<R(X,Y)Z,W> + <R(X,Y)W,Z> = 0) in outer symbols, but
(4) unchanged when inner and outer symbols are exchanged (<R(X,Y)Z,W> = <R(Z,W)X,Y>).
The fourth property is the most difficult, its proof requiring use of the other three &endash; and leading to the diagram as an aid to its following. Says Milnor: Formula (2) asserts that the sum of the quantities at the vertices of shaded triangle W is zero. Similarly (making use of (1) and (3)) the sum of the vertices of each of the other shaded triangles is zero. Adding these identities for the top two shaded triangles, and subtracting the identities for the bottom ones, this means that twice the top vertex minus twice the bottom vertex is zero. This proves (4), and completes the proof.
With Sedenion-based box-kites, of course, the shaded triangles comprise the sails, and the products along each of their edges are zero; the terms at opposed ends of struts, meanwhile, obey a different relation from those connected to each other in a sail &endash; not their sums, but their differences, must be attended to. Can a small number of "symmetry breaks" and/or "categorical removes" serve to link these radically different uses of the box-kite diagram? ...".
you can expand, by a sort of tensegrity transformation, the onarhedron into a cuboctahedron. The cuboctahedron is a central figure
expandable to a 24-cell. The 24-cell can be expanded into a 240-vertex real 8-dim Witting polytope. (The real Witting polytope lives in 8-dim real space, while the complex Witting polytope lives in 4-dim complex space.) The 240 vertices of the real Witting polytope correspond to half of the 480 octonion products. The real Witting polytope is the vertex figure of an E8 lattice. There are 7 distinct real Witting polytopes and 7 distinct E8 lattices each sharing some vertices with some of the others. All 7 real Witting polytopes, taken together, have 480 distinct vertices, each representing a distinct octonion product. The 7 real Witting polytopes, and 7 E8 lattices, correspond to the octonion imaginaries, which is where we started the reflexive loop: octonion imaginaries; onarhedron; cuboctahedron; 24-cell; real Witting polytope; E8 lattices; octonion imaginaries; ...
Just as 8-dimensional E8 lattices can be formed by Witting polytopes, and 4-dimensional space can be filled by 24-cells, and 2-dimensional space can be filled by hexagons, triangles, or squares, so 3-dimensional space can be filled by octahedra and cuboctahedra, and Onar Aam has shown that onarhedra and cuboctahedra consistenly fit together to form an
Since cuboctahedra plus octahedra fill 3-dim space, you can use cuboctahedra plus onarhedra to fill 3-dim space so that both the cuboctahedra and onarhedra are octonionic: K---k---E---I---i---K---J---j---E---i---I---k---J---E---j---K---I | | + | | + | | + | | + | j j+++i+++k k+++E+++K K+++J+++i i+++I+++E | | + | | + | | + | | + | I---k---J---E---j---K---I---J---k---i---j---I---K---E---k---j---i | + | | + | | + | | + | | K+++J+++i i+++I+++E E+++j+++J J+++k+++I I | + | | + | | + | | + | | j---I---K---E---k---j---i---J---K---k---E---I---i---K---J---j---E | | + | | + | | + | | + | J J+++k+++I I+++K+++j j+++i+++k k+++E+++K | | + | | + | | + | | + | E---I---i---K---J---j---E---i---I---k---J---E---j---K---I---J---k | + | | + | | + | | + | | j+++i+++k k+++E+++K K+++J+++i i+++I+++E E | + | | + | | + | | + | | J---E---j---K---I---J---k---i---j---I---K---E---k---j---i---J---K This is a chessboard lattice of onarhedra and cuboctahedra. The 2D cross made of +'es are the onarhedra and the "empty" squares next to them are "flattened" cuboctahedra. If you deform the above lattice by contracting the cuboctahedra so that the similar imaginaries on the opposite sides merge, you get two interwovern onarhedral lattices. You can also use onarhedra to get triangular tilings that may be similar to tilings of tetrahedra and truncated tetrehedra: i___E___J___I___j___k___K___i \*/ \*/ \*/ \*/ \*/ \*/ \*/ \ I___j___k___K___i___E___J___I / \*/ \*/ \*/ \*/ \*/ \*/ \*/ k___K___i___E___J___I___j___k \*/ \*/ \*/ \*/ \*/ \*/ \*/ \ E___J___I___j___k___K___i___E / \*/ \*/ \*/ \*/ \*/ \*/ \*/ I___j___k___K___i___E___J___I \*/ \*/ \*/ \*/ \*/ \*/ \*/ \ K___i___E___J___I___j___k___K The * is inside the octonionic triangles, those pointing downward. The triangles pointing upward are not associative, but they can be made into co-associative tetrahedra by adding an appropriate imaginary above them. This tiling is self-similar in that, if you replace each triangle with its corresponding imaginary, then you get exactly the same tile. The cuboctahedron-onarhedron tiling uses the interior coassociative squares of onarhedra, whereas the triangular tiling uses the exterior associative triangles.
is another manifestation of the reflexive structure of Octonions. If you look only at the onarhedral part of the space-filling cuboctahedron-onarhedron tiling, you get a tiling by onarhedra that does not fill all of 3-dimensional space, but does extend throughout 3-dimensional space:
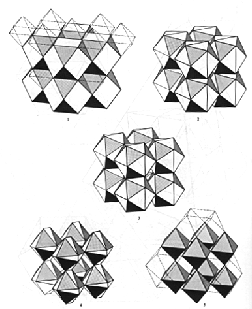
In a paper astro-ph/9801276 (written in the context of large-scale structure of our universe) E. Battaner shows how such a non-filling tiling by octahedra can be subdivided into a similar non-filling tiling by smaller (1/3 length scale) octahedra, with 7 small octahedra within each larger octahedron, as shown in this image from his paper:
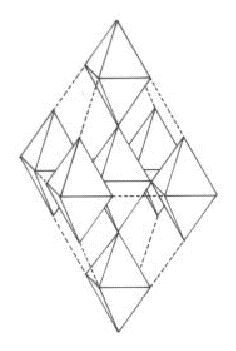
The octahedral fractal structure of E. Battaner also forma an onarhedral fractal structure. Just as the faces, etc., of the onarhedron correspond to the 7 octonion imaginaries, the 7 subonarhedra (one at each vertex plus one in the middle) also correspond to the 7 octonion imaginaries. Further, the 3 smaller subonarhedra on each axis of a larger onarhedron correspond to an associative triangle. Within each large onarhedron, in addition to the 7 small onarhedra, there are 8 small cuboctahedra. The 7 small onarhedra correspond to the 7 octonion imaginaries, and the 8 small cuboctahedra to the 8 additional sedenion imaginaries. They are related to the last Hopf fibration of spheres: S7 to S15 to S8
This page is a corrected and updated (29 January 1998) version of a talk given at Corvallis in April 1997. For more about cross products, octonions and related matters, see pages about Octonions, about Clifford Algebras, and about Sedenions. For applications to the D4-D5-E6 model of physics, see From Sets to Quarks, particularly Chapter 2.
n Total Spinor Dimension Dimension 0 1 2^0 = 1= 1x1 1 1 1 1 2^1 = 2= 1+1 1 2 1 2 1 2^2 = 4= 2x2 2 = 1+1 3 1 3 3 1 2^3 = 8= 4+4 2 4 1 4 6 4 1 2^4 = 16= 4x4 4 = 2+2 5 1 5 10 10 5 1 2^5 = 32=16+16 4 6 1 6 15 20 15 6 1 2^6 = 64= 8x8 8 = 4+4 7 1 7 21 35 35 21 7 1 2^7 = 128=64+64 8 8 1 8 28 56 70 56 28 8 1 2^8 = 256=16x16 16 = 8+8 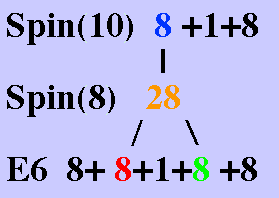
are 9x8 = 72-dimensional: O1 O4 O5 O7 O2 O6 O8 O9 O3
are 3x8 + 3x1 = 27-dimensional: Re(O1) O4 O5 O4* Re(O2) O6 O5* O6* Re(O3) With symmetric product AB = (1/2)(AB + BA) they form the exceptional Jordan algebra J3(O), which is related to the 27-dimensional MacroSpace of the D4-D5-E6-E7-E8 VoDou Physics model.
are 3x8 + 3x7 = 45-dimensional: Im(O1) O4 O5 -O4* Im(O2) O6 -O5* -O6* Im(O3) Under the antisymmetric product AB = (1/2)(AB - BA) the matrices do not close. If we restrict ourselves to traceless matrices and use the traceless product AB = (1/2)(AB - BA - Tr((AB - BA)) thus reducing the space to 45-7 = 38 dimensions, we get closure: Im(O1) O4 O5 -O4* Im(O2) O6 -O5* -O6* - but due to nonassociativity the Jacobi identity is not satisfied. If we add the 14-dimensional octonion derivation algebra G2, we get satisfy the Jacobi identity: Im(O1) O4 O5 -O4* Im(O2) O6 -O5* -O6* - x G2 The resulting Lie algebra is 38+14 = 52-dimensional F4. Note that 52-dim F4 has a 28-dim subgroup, Spin(8),
Im(O1) - - - Im(O2) - - - - x G2 that is a fibration product of G2 and two copies of Im(O).
can be built from 52-dim AntiHermitian traceless 3x3 F4 by adding the 26-dim Hermitian traceless part of J3(O): Im(O1) O4 O5 -O4* Im(O2) O6 -O5* -O6* - x G2 x Re(O1) O4 O5 O4* Re(O2) O6 O5* O6* -
has graded dimensions: grade 0 1 2 3 4 5 6 7 8 dimension 1 8 28 56 70 56 28 8 1 *-grade 8 7 6 5 4 3 2 1 0 The Hodge star * map takes the 70-dimensional 4-vector space into itself. Therefore, the 70-dimensional 4-vector space splits into two 35-dimensional parts that are interchanged by the Hodge star * map. 256-dimensional Cl(8) can be represented by a 16x16 real matrix algebra. The numbers refer to the grade in Cl(8) of the matrix entry. 0 2 2 2 2 2 2 2 7 5 5 5 5 5 5 5 4 4 2 2 2 2 2 2 5 7 5 5 5 5 5 5 4 4 4 2 2 2 2 2 5 5 7 5 5 5 5 5 4 4 4 4 2 2 2 2 5 5 5 7 5 5 5 5 4 4 4 4 4 2 2 2 5 5 5 5 7 5 5 5 4 4 4 4 4 4 2 2 5 5 5 5 5 7 5 5 4 4 4 4 4 4 4 2 5 5 5 5 5 5 7 5 4 4 4 4 4 4 4 4 5 5 5 5 5 5 5 7 * 1 3 3 3 3 3 3 3 4 4 4 4 4 4 4 4 3 1 3 3 3 3 3 3 6 4 4 4 4 4 4 4 3 3 1 3 3 3 3 3 6 6 4 4 4 4 4 4 3 3 3 1 3 3 3 3 6 6 6 4 4 4 4 4 3 3 3 3 1 3 3 3 6 6 6 6 4 4 4 4 3 3 3 3 3 1 3 3 6 6 6 6 6 4 4 4 3 3 3 3 3 3 1 3 6 6 6 6 6 6 4 4 3 3 3 3 3 3 3 1 6 6 6 6 6 6 6 8
To make connection with observable physics in our regime, far below the Planck energy of 10^19 GeV, the 8-dimensional nonassociative octonionic spacetime of Cl(8) must be reduced to a 4-dimensional associative submanifold.
Since the more realistic Minkowski Physical SpaceTime with -+++ Signature has Quaternionic Structure, a useful Clifford path is this Clifford Path that is Quaternionic from Cl(3,5) through Cl(2,4) to Cl(1,3) and from Cl(2,6) through Cl(2,5) and Cl(2,4) to Cl(1,3):
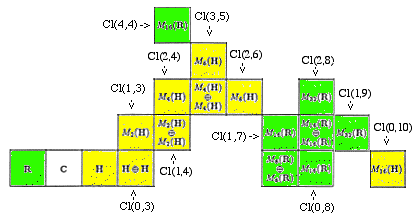
In the D4-D5-E6-E7-E8 VoDou Physics model, D5 is taken to be SL(2,O) = Spin(1,9), and spinors are R16 + R16 of Cle(1,9) = Cl(1,8),
where Cle(p,q) = Cl(p,q-1) and Cle(p,0) = Cl(0,p-1)
The D4 can initially be taken to be Spin(0,8), or, with the same Clifford algebra, Spin(1,7) or Spin(4,4). Dimensional Reduction from 8 to 4 dimensions introduces the subspace substructure of Quaternionic Physical SpaceTime in Octonionic Vector Space.
The (1,7)-dimensional RP1 x S7 = S1 x S7 = U(1) x S7 spacetime of the D4-D5-E6-E7-E8 VoDou Physics model prior to dimensional reduction can be represented by Quaternionic Projective 2-space QP2.
Atiyah and Berndt say in their paper Projective Planes, Serveri Varieties, and Spheres, math.DG/0206135, the S1 x S7 considered as QP2 breaks down into two parts:
Therefore, dimensional reduction changes Real M(16,R) of Cl(0,8), Cl(1,7), and Cl(4,4) to the Quaternionic M(8,Q) of Cl(2,6) or Cl(3,5).
Cl(2,6) = M(8,Q) Spin*(8) = Spin(2,6) Cl(2,5) = M(4,Q)+M(4,Q) Spin(2,5) Cl(2,4) = M(4,Q) Spin(2,4) = SU(2,2)
Spin(2,4) = SU(2,2) is the connected component with identity of the Conformal(1,3) Group of Minkowski SpaceTime. Since both 1+1 and 3+1 are even, Conformal(1,3) has 4 components, not 2 components as for Conformal(p,q) where p+1 and/or q+1 are odd. U(2,2) = U(1)xSU(2,2) corresponds to compact U(4) = U(1)xSU(4), the compact isotropy space of the 12-dimensional rank-2 symmetric spaces Spin*(8) / U(4) (non-compact) and Spin(8) / U(4) (compact).
Cl(1,4) = M(2,Q)+M(2,Q) Spin(1,4) = Sp(1,1)
Spin(1,4) is the connected component with identity of the deSitter Group of Minkowski SpaceTime. Although the MacDowell-Mansouri mechanism, as originally formulated, produced gravity from anti-deSitter Spin(2,3) = Spin(0,5) = Sp(2), this Quaternionic chain uses, instead of Sp(2), Sp(1,1) = Spin(1,4).
Cl(1,3) = M(2,Q) Spin(1,3)
Spin(1,3) is the connected component with identity of the Lorentz Group of Minkowski SpaceTime.
Cl(0,3) = Q+Q Spin(0,3) = Sp(1) = SU(2) = S3 Cl(0,2) = Q Spin(0,2) = U(1) Cl(0,1) = C Spin(0,1) = Z2 Cl(0,0) = R Spin(0,0) = I
(Much of the above is taken from Ian Porteous's book Clifford Algebras and the Classical Groups (Cambridge 1995).)
The path from Cl(1,7) through Cl(2,4) to Cl(1,3) can also be seen from the viewpoint of spinor fermions of the D4-D5-E6-E7-E8 VoDou Physics model:
Half-Spinors for 16x16 real matrix Cl(1,7) are 1x8 real column vectors
which correspond to first-generation fermion particles:
Octonion Fermion
Basis Element Particle
1 e-neutrino
i red up quark
j green up quark
k blue up quark
I red down quark
J green down quark
K blue down quark
E electron
Full Spinors for 4x4 quaternion matrix Cl(2,4) are
1x4 quaternion column vectors:
q1
q2
q3
q4
which are reducible to two Half-Spinor mirror image
1x2 quaternion column vectors:
q+1
q+2
q-1
q-2
Since each of these is a 4-dimensional quaternion,
if you let the q+ be fermion particles
and the q- be fermion antiparticles,
you have
4 fermion particles for q+1, which can be taken to
be electron, red up quark, blue up quark, and green up quark,
and
4 fermion particles for q+2, which can be taken to
be neutrino, red down quark, blue down quark, and green down quark,
and
4 fermion antiparticles for q-1, which can be taken to
be positron, red up antiquark, blue up antiquark, and green up antiquark,
and
4 fermion antiparticles for q-2, which can be taken to be antineutrino,
red down antiquark, blue down antiquark, and green down antiquark,
so that the quaternionic spinors of Cl(2,4) correspond directly
to the fermions of the real spinors of Cl(1,7).
For Cl(1,3) the 2x2 quaternionic matrices have Full Spinors
that are 1x2 quaternion column vectors.
Each Half-Spinor space is one quaternion variable,
which has a 1-2 correspondence with first generation fermions,
and also corresponds 1-1 with the (1,3) vector space of
physical Minkowski spacetime,
resulting in a quaternionic version of triality (diluted by
the 1-2 nature of the fermion correspondence) that is
related to the reducibility of the D2 Lie algebra Spin(1,3).
This page is a corrected and updated (January 2001, September 2003)
version of a talk given at Corvallis in April 1997.
Tony's Home
......