The quark content of a charged pion is a quark - antiquark pair: either Up plus antiDown or Down plus antiUp. Experimentally, its mass is about 139.57 MeV.
The quark is a Naked Singularity Kerr-Newman Black Hole, with electromagnetic charge e and spin angular momentum J and constituent mass M 312 MeV, such that e^2 + a^2 is greater than M^2 (where a = J / M).The antiquark is a also Naked Singularity Kerr-Newman Black Hole, with electromagnetic charge e and spin angular momentum J and constituent mass M 312 MeV, such that e^2 + a^2 is greater than M^2 (where a = J / M).
According to General Relativity, by Robert M. Wald (Chicago 1984) page 338 [Problems] ... 4. ...:
"... Suppose two widely separated Kerr black holes with parameters ( M1 , J1 ) and ( M2 , J2 ) initially are at rest in an axisymmetric configuration, i.e., their rotation axes are aligned along the direction of their separation.Assume that these black holes fall together and coalesce into a single black hole.
Since angular momentum cannot be radiated away in an axisymmetric spacetime, the final black hole will have momentum J = J1 + J2. ...".
The neutral pion produced by the quark - antiquark pair would have zero angular momentum, thus reducing the value of e^2 + a^2 to e^2 .
For fermion electrons with spin 1/2, 1 / 2 = e / M (see for example Misner, Thorne, and Wheeler, Gravitation (Freeman 1972), page 883) so that M^2 = 4 e^2 is greater than e^2 for the electron. In other words, the angular momentum term a^2 is necessary to make e^2 + a^2 greater than M^2 so that the electron can be seen as a Kerr-Newman naked singularity.
Since the magnitude of electromagnetic charge of each quarks or antiquarks less than that of an electron, and since the mass of each quark or antiquark (as well as the pion mass) is greater than that of an electron, and since the quark - antiquark pair (as well as the pion) has angular momentum zero, the quark - antiquark pion has M^2 greater than e^2 + a^2 = e^2.
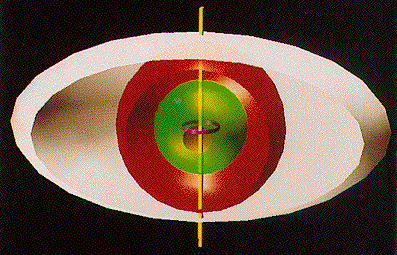
Therefore, the pion itself is a normal Kerr-Newman Black Hole with Outer Event Horizon = Ergosphere at r = 2M ( the Inner Event Horizon is only the origin at r = 0 ) as shown in this image

from Black Holes - A Traveller's Guide, by Clifford Pickover (Wiley 1996) in which the Ergosphere is white, the Outer Event Horizon is red, the Inner Event Horizon is green, and the Ring Singularity is purple. In the case of the pion, the white and red surfaces coincide, and the green surface is only a point at the origin.
According to section 3.6 of Jeffrey Winicour's 2001 Living Review of the Development of Numerical Evolution Codes for General Relativity (see also a 2005 update):
"... The black hole event horizon associated with ... slightly broken ... degeneracy [ of the axisymmetric configuration ]... reveals new features not seen in the degenerate case of the head-on collision ... If the degeneracy is slightly broken, the individual black holes form with spherical topology but as they approach, tidal distortion produces two sharp pincers on each black hole just prior to merger.... Tidal distortion of approaching black holes ...
... Formation of sharp pincers just prior to merger ..
... toroidal stage just after merger ...
At merger, the two pincers join to form a single ... toroidal black hole.
The inner hole of the torus subsequently [ begins to] close... up (superluminally) ... [ If the closing proceeds to completion, it ]... produce[s] first a peanut shaped black hole and finally a spherical black hole. ...".
In the physical case of quark and antiquark forming a pion, the toroidal black hole remains a torus. The torus is an event horizon and therefore is not a 2-spacelike dimensional torus, but is a (1+1)-dimensional torus with a timelike dimension.
The effect is described in detail in Robert Wald's book General Relativity (Chicago 1984). It can be said to be due to extreme frame dragging, or to timelike translations becoming spacelike as though they had been Wick rotated in Complex SpaceTime.
As Hawking and Ellis say in The LargeScale Structure of Space-Time (Cambridge 1973):
"... The surface r = r+ is ... the event horizon ... and is a null surface ...
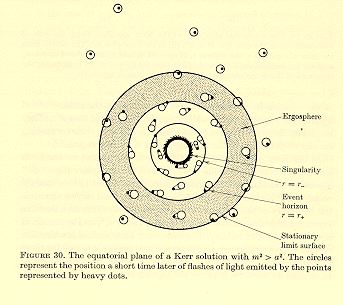
... On the surface r = r+ .... the wavefront corresponding to a point on this surface lies entirely within the surface. ...".
A (1+1)-dimensional torus with a timelike dimension can carry a Sine-Gordon Breather, and the soliton and antisoliton of a Sine-Gordon Breather correspond to the quark and antiquark that make up the pion.
Sine-Gordon Breathers are described by Sidney Coleman in his Erica lecture paper Classical Lumps and their Quantum Descendants (1975), reprinted in his book Aspects of Symmetry (Cambridge 1985), where Coleman writes the Lagrangian for the Sine-Gordon equation as ( Coleman's eq. 4.3 ):
and Coleman says:
"... We see that, in classical physics, B is an irrelevant parameter: if we can solve the sine-Gordon equation for any non-zero B, we can solve it for any other B. The only effect of changing B is the trivial one of changing the energy and momentum assigned to a given soluition of the equation. This is not true in quantum physics, becasue the relevant object for quantum physics is not L but [ eq. 4.4 ]L / hbar = (1 / ( B^2 hbar ) ) ( (1/2) (df)^2 + A ( cos( f ) - 1 ) ) An other way of saying the same thing is to say that in quantum physics we have one more dimensional constant of nature, Planck's constant, than in classical physics. ... the classical limit, vanishingf hbar, is exactly the same as the small-coupling limit, vanishing B ... from now on I will ... set hbar equal to one. ...
... the sine-Gordon equation ...[ has ]... an exact periodic solution ...[ eq. 4.59 ]...
f( x, t ) = ( 4 / B ) arctan( ( n sin( w t ) / cosh( n w x )) where [ eq. 4.60 ] n = sqrt( A - w^2 ) / w and w ranges from 0 to A. This solution has a simple physical interpretation ... a soliton far to the left ...[ and ]... an antisoliton far to the right. As sin( w t ) increases, the soliton and antisoliton mover farther apart from each other. When sin( w t ) passes thrpough one, they turn around and begin to approach one another. As sin( w t ) comes down to zero ... the soliton and antisoliton are on top of each other ... when sin( w t ) becomes negative .. the soliton and antisoliton have passed each other. ...[
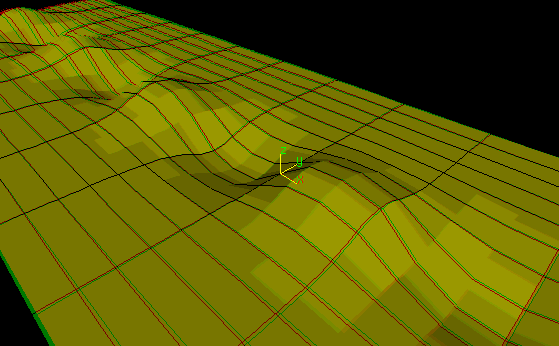
This stereo image of a Sine-Gordon Breather was generated by the program 3D-Filmstrip for Macintosh by Richard Palais. You can see the stereo with red-green or red-cyan 3D glasses. The program is on the WWW at http://rsp.math.brandeis.edu/3D-Filmstrip. The Sine-Gordon Breather is confined in space (y-axis) but periodic in time (x-axis), and therefore naturally lives on the (1+1)-dimensional torus with a timelike dimension of the Event Horizon of the pion. ...]... Thus, Eq. (4.59) can be thought of as a soliton and an antisoliton oscillation about their common center-of-mass. For this reason, it is called 'the doublet [ or Breather ] solution'. ... the energy of the doublet ...[ eq. 4.64 ]
E = 2 M sqrt( 1 - ( w^2 / A ) ) where [ eq. 4.65 ] M = 8 sqrt( A ) / B^2 is the soliton mass. Note that the mass of the doublet is always less than twice the soliton mass, as we would expect from a soltion-antisoliton pair. ... Dashen, Hasslacher, and Neveu ... Phys. Rev. D10, 4114; 4130; 4138 (1974). A pedagogical review of these methods has been written by R. Rajaraman ( Phys. Reports 21, 227 (1975 ... Phys. Rev. D11, 3424 (1975) ...[ Dashen, Hasslacher, and Neveu found that ]... there is only a single series of bound states, labeled by the integer N ... The energies ... are ... [ eq. 4.82 ]
E_N = 2 M sin( B'^2 N / 16 ) where N = 0, 1, 2 ... < 8 pi / B'^2 , [ eq. 4.83 ]
B'^2 = B^2 / ( 1 - ( B^2 / 8 pi )) and M is the soliton mass. M is not given by Eq. ( 4.675 ), but is the soliton mass corrected by the DHN formula, or, equivalently, by the first-order weak coupling expansion. ... I have written the equation in this form .. to eliminate A, and thus avoid worries about renormalization conventions. Note that the DHN formula is identical to the Bohr-Sommerfeld formula, except that B is replaced by B'. ... Bohr and Sommerfeld['s] ... quantization formula says that if we have a one-parameter family of periodic motions, labeled by the period, T, then an energy eigenstate occurs whenever [ eq. 4.66 ]
[ Integral from 0 to T ]( dt p qdot = 2 pi N, where N is an integer. ... Eq.( 4.66 ) is cruder than the WKB formula, but it is much more general; it is always the leading approximation for any dynamical system ... Dashen et al speculate that Eq. ( 4.82 ) is exact. ...
the sine-Gordon equation is equivalent ... to the massive Thirring model. This is surprising, because the massive Thirring model is a canonical field theory whose Hamiltonian is expressedin terms of fundamental Fermi fields only. Even more surprising, when B^2 = 4 pi , that sine-Gordon equation is equivalent to a free massive Dirac theory, in one spatial dimension. ... Furthermore, we can identify the mass term in the Thirring model with the sine-Gordon interaction, [ eq. 5.13 ]
M = - ( A / B^2 ) N_m cos( B f ) .. to do this consistently ... we must say [ eq. 5.14 ]
B^2 / ( 4 pi ) = 1 / ( 1 + g / pi ) ....[where]... g is a free parameter, the coupling constant [ for the Thirring model ]... Note that if B^2 = 4 pi , g = 0 , and the sine-Gordon equation is the theory of a free massive Dirac field. ... It is a bit surprising to see a fermion appearing as a coherent state of a Bose field. Certainly this could not happen in three dimensions, where it would be forbidden by the spin-statistics theorem. However, there is no spin-statistics theorem in one dimension, for the excellent reason that there is no spin. ... the lowest fermion-antifermion bound state of the massive Thirring model is an obvious candidate for the fundamental meson of sine-Gordon theory. ... equation ( 4.82 ) predicts that all the doublet bound states disappear when B^2 exceeds 4 pi . This is precisely the point where the Thirring model interaction switches from attractive to repulsive. ... these two theories ... the massive Thirring model .. and ... the sine-Gordon equation ... define identical physics. ... I have computed the predictions of ...[various]... approximation methods for the ration of the soliton mass to the meson mass for three values of B^2 : 4 pi (where the qualitative picture of the soliton as a lump totally breaks down), 2 pi, and pi . At 4 pi we know the exact answer ... I happen to know the exact answer for 2 pi, so I have included this in the table. ...
Method B^2 = pi B^2 = 2 pi B^2 = 4 pi Zeroth-order weak coupling expansion eq2.13b 2.55 1.27 0.64 Coherent-state variation 2.55 1.27 0.64 First-order weak coupling expansion 2.23 0.95 0.32 Bohr-Sommerfeld eq4.64 2.56 1.31 0.71 DHN formula eq4.82 2.25 1.00 0.50 Exact ? 1.00 0.50...[eq. 2.13b ] E = 8 sqrt(A) / B^2 ...[ is the ]... energy of the lump ... of sine-Gordon theory ... frequently called 'soliton...' in the literature ... [ Zeroth-order is the classical case, or classical limit. ] ...
... Coherent-state variation always gives the same result as the ... Zeroth-order weak coupling expansion ... .
The ... First-order weak-coupling expansion ... explicit formula ... is ( 8 / B^2 ) - ( 1 / pi ). ...".
Note that,
using the VoDou Physics constituent mass of the Up and Down quarks and antiquarks, about 312.75 MeV, as the soliton and antisoliton masses,and setting B^2 = pi
and using the DHN formula,
the mass of the charged pion is calculated to be ( 312.75 / 2.25 ) MeV = 139 MeV
which is in pretty good agreement with the experimental value of about 139.57 MeV.
Because B^2 = pi is where the First-order weak coupling expansion substantially coincides with the ( probably exact ) DHN formula. In other words,
Near the end of his article, Coleman expressed "Some opinions":
"... This has been a long series of physics lectures with no reference whatsoever to experiment. This is embarrassing.... Is there any chance that the lump will be more than a theoretical toy in our field? I can think of two possiblities.
One is that there will appear a theory of strong-interaction dynamics in which hadrons are thought of as lumps, or, ... as systems of quarks bound into lumps. ... I am pessimistic about the success of such a theory. ... However, I stand ready to be converted in a moment by a convincing computation.
The other possibility is that a lump will appear in a realistic theory ... of weak and electromagnetic interactions ... the theory would have to imbed the U(1)xSU(2) group ... in a larger group without U(1) factors ... it would be a magnetic monopole. ...".
As to his second stated possibility, very massive monopoles related to SU(5) GUT are still within the realm of possible future experimental discoveries.
Further material about the sine-Gordon doublet Breather and the massive Thirring equation can be found in the book Solitons and Instantons (North-Holland 1982,1987) by R. Rajaraman, who writes:
"... the doublet or breather solutions ... can be used as input into the WKB method. ... the system is ... equivalent to the massive Thirring model, with the SG soliton state identifiable as a fermion. ... Mass of the quantum soliton ... will consist of a classical term followed by quantum corrections. The energy of the classical soliton ... is ... [ eq. 7.3 ]E_cl[f_sol] = 8 m^3 / L The quantum corrections ... to the 'soliton mass' ... is finite as the momentum cut-off goes to infinity and equals ( - m / pi ). Hence the quantum soliton's mass is [ eq. 7.10 ]
M_sol =( 8 m^3 / L ) - ( m / pi ) +O(L). The mass of the quantum antisoliton will be, by ... symmetry, the same as M_sol. ...
The doublet solutions ... may be quantised by the WKB method. ... we see that the coupling constant ( L / m^2 ) has been replaced by a 'renormalised' coupling constant G ... [ eq. 7.24 ]
G = ( L / m^2 ) / ( 1 - ( L / 8 pi m^2 )) ... as a result of quantum corrections. ... the same thing had happened to the soliton mass in eq. ( 7.10 ). To leading order, we can write [ eq. 7.25 ]
M_sol = ( 8 m^3 / L ) - ( m / pi ) = 8 m / G ... The doublet masses ... bound-state energy levels ... E = M_N, where ... [ eq. 7.28 ]
M_N = ( 16 m / G ) sin( N G / 16 ) ; N = 1, 2, ... < 8 pi / G Formally, the quantisation condition permits all integers N from 1 to oo , but we run out of classical doublet solutions on which these bound states are based when N > 8 pi / G . ... The classical solutions ... bear the same relation to the bound-state wavefunctionals ... that Bohr orbits bear to hydrogen atom wavefunctions. ...
Coleman ... show[ed] explicitly ... the SG theory equivalent to the charge-zero sector of the MT model, provided ... L / 4 pi m^2 = 1 / ( 1 + g / pi )
...[ where in Coleman's work set out above such as his eq. ( 5.14 ) , B^2 = L / m^2 ]...
Coleman ... resurrected Skyrme's conjecture that the quantum soliton of the SG model may be identified with the fermion of the MT model. ... ".
WHAT ABOUT THE NEUTRAL PION?
The quark content of the charged pion is u_d or d_u , both of which are consistent with the sine-Gordon picture. Experimentally, its mass is 139.57 Mev. The neutral pion has quark content (u_u + d_d)/sqrt(2) with two components, somewhat different from the sine-Gordon picture, and a mass of 134.96 Mev. The effective constituent mass of a down valence quark increases (by swapping places with a strange sea quark) by about DcMdquark = (Ms - Md) (Md/Ms)2 aw V12 = = 312x0.25x0.253x0.22 Mev = 4.3 Mev. Similarly, the up quark color force mass increase is about DcMuquark = (Mc - Mu) (Mu/Mc)2 aw V12 = = 1777x0.022x0.253x0.22 Mev = 2.2 Mev. The color force increase for the charged pion DcMpion± = 6.5 Mev. Since the mass Mpion± = 139.57 Mev is calculated from a color force sine-Gordon soliton state, the mass 139.57 Mev already takes DcMpion± into account. For pion0 = (u_u + d_d)/ sqrt 2 , the d and _d of the the d_d pair do not swap places with strange sea quarks very often because it is energetically preferential for them both to become a u_u pair. Therefore, from the point of view of calculating DcMpion0, the pion0 should be considered to be only u_u , and DcMpion0 = 2.2+2.2 = 4.4 Mev. If, as in the nucleon, DeM(pion0-pion±) = -1 Mev, the theoretical estimate is DM(pion0-pion±) = DcM(pion0-pion±) + DeM(pion0-pion±) = = 4.4 - 6.5 -1 = -3.1 Mev, roughly consistent with the experimental value of -4.6 Mev.
In his book Quaternionic Quantum Mechanics and Quantum Fields ((Oxford 1995), Stephen L. Adler says at pages 50-52, 561:
"... If the multiplication is associative, as in the complex and quaternionic cases, we can remove parentheses in ... Schroedinger equation dynamics ... to conclude that ... the inner product < f(t) | g(t) > ... is invariant ... this proof fails in the octonionic case, and hence one cannot follow the standard procedure to get a unitary dynamics. ...[sothere is a]... failure of unitarity in octonionic quantum mechanics...".
Conventionally, creation of the particles in our universe occurred during inflation with unitarity and energy conservation being due to an inflaton field that is addition to the fields we now observe in the Standard Model plus Gravity.
In my VoDou physics model, our present 4-dimensional physical spacetime freezes out from a high-energy 8-dimensional octonionic spacetime due to selection of a preferred quaternionic subspacetime. A question is whether the dimensional reduction occurs at the initial Big Bang beginning of inflation or continues through inflation to its end.
The non-associative structure of octonions manifests itself in interesting ways
7-dim S7 EXPANDS TO S7 x G2 x S7: Spin(8) Lie Algebra
/ \
|
|
\ /
7 Imaginary Octonions i j k E I J K
/ \
|
|
\ /
7 (Associative Triangles+1) x 16 (4 signs) = 112
/ \
/ / \ \
7 E8 Lattices | 224 + 16 = 240 Units
\ | / x2 (RL)
\ \ / / ||
\ / 480 Octonion Products
7 Coassociative Squares x 16 (4 signs) = 112
and a correspondence between each of the 7 imaginary octonions and each of 7 Onarhedra/Heptavertons
E
/ \
/ \
J-----j E j
J I---j / | | \ |/
I --> / \ --> | | --> i | I | i = J--I--k
i---K k---E \ | | / /|
K-----k K i
\ /
\ /
E
E
/ \
/ \
K-----k E k
j J---i / | | \ |/
J --> / \ --> | | --> j | J | j = K--J--i
I---K k---E \ | | / /|
I-----i I i
\ /
\ /
E
E
/ \
/ \
I-----i E i
J K---i / | | \ |/
K --> / \ --> | | --> k | K | k = I--K--j
I---k j---E \ | | / /|
J-----j J k
\ /
\ /
E
k
/ \
/ \
I-----J k J
I J---j / | | \ |/
i --> / \ --> | | --> j | i | j = I--i--E
E---i K---k \ | | / /|
K-----E K j
\ /
\ /
k
k
/ \
/ \
J-----I k I
J K---k / | | \ |/
j --> / \ --> | | --> i | j | i = J--j--E
E---j I---i \ | | / /|
K-----E K i
\ /
\ /
k
i
/ \
/ \
K-----J i J
K I---i / | | \ |/
k --> / \ --> | | --> j | k | j = K--k--E
E---k J---j \ | | / /|
I-----E I j
\ /
\ /
i
I
/ \
/ \
J-----k I k
j I---J / | | \ |/
E --> / \ --> | | --> i | E | i = J--E--j
i---k K---E \ | | / /|
K-----j K i
\ /
\ /
I
Just as each of the 7 imaginary octonions correspond, in my VoDou physics model, to the 7 types of charged fermions (electron; red, blue, green up quarks; red, blue, green down quarks),
each Onarhedron/Heptaverton corresponds to a charge-neutral set of all 7 charged fermions.
Consider that the initial Big Bang produced a particle-antiparticle pair of the 7 charged fermions, plus the 8th fermion (neutrino) corresponding to the real number 1.
The paper gr-qc/0007006 by Paola Zizzi shows that "... during inflation, the universe can be described as a superposed state of quantum ... [ qubits ]. The self-reduction of the superposed quantum state is ... reached at the end of inflation ...[at]... the decoherence time ... [ Tdecoh = 10^9 Tplanck = 10^(-34) sec ] ... and corresponds to a superposed state of ... [ 10^19 = 2^64 qubits ]. ... ... This is also the number of superposed tubulins-qubits in our brain ... leading to a conscious event. ...".
The number of doublings (also known as e-foldings) is also estimated by om astro-ph/0307459, by Banks and Fischler, who say: "... If the present acceleration of the universe is due to an asymptotically deSitter universe with small cosmological constant, then the number of e-foldings during inflation is bounded. ... The essential ingredient is that because of the UV-IR connection, entropy requires storage space. The existence of a small cosmological constant restricts the available storage space. ... We obtain the upper bound ... N_e = 85 ... where we took [the cosmological constant] /\ to be of O(10^(-3) eV ). For the sake of comparison, the case k = 1/3 [ corresponding to the equation of state for a radiation-dominated fluid, such as the cosmic microwave background ] yields ... N_e= 65 ... This value for the maximum number of e-foldings is close to the value necessary to solve the "horizon problem".
If at each of the 64 doubling stages of Zizzi inflation the 2 particles of such a pair produced 8+8 = 16 fermions,
then at the end of inflation such a non-unitary octonionic process would have produced about 2 x 16^64 = 4 x (2^4)^64 = 4 x 2^256 = 4 x 10^77 fermion particles.
The figure of 4 x 10^77 is similar number of particles estimated by considering the initial fluctuation to be a Planck mass Black Hole and the 64 doublings to act on such Black Holes (which process can also be considered due to octonionic non-associativity non-unitarity).
Bert Schroer said in a comment on Peter's blog entry "Hype from the Swampland":
"... The local operator algebras in QFT are all of one kind, i.e. if you have seen one, you know them all (just like points in geometry) and the richness of QFT, including its inner symmetries and its (noncompact) spacetime symmetries, is all contained in the relative position of a finite number of copies of this "monade" (explicitly: hyperfinite Type III_1 von Neumann algebras ...) ... the monades used here lead to modular inclusions and they lead to spacetime ... The concepts and mathematics are clear and rigorous ... the very nature of those above mentioned monade algebras already incorporates the vacuum polarization and thermal properties in their algebraic structure (these algebras have no pure states at all!). They are the heart of local quantum physics (much more basic than the use of particular field coordinatizations) ...".
My physics model, instead of hyperfinite III_1, uses Clifford algebra periodicity ideas to try to generalize hyperfinite II_1, as follows:
If you consider that hyperfinite II_1 to be based on 2x2 complex matrices, and you consider them to be the complex Clifford algebra Cl(2;C), then, since complex Clifford periodicity is 2, you have
Cl(2N;C) = Cl(2;C) x ...(N times tensor product)... x Cl(2;C)
It seems to me that the underlying reason why you can take the unions and do the completion without problems is that everything factors down to Cl(2;C) structure, and no new strange structure is introduced no matter how high is the dimension you are dealing with.
Since the Cl(2;C) each look like fermionic creation/annihilation operators, you get a nice picture of fermions.
I am trying to repeat the same process with real Clifford algebras. There, periodicity is 8 and you have (I am ignoring signature here for brevity)
Cl(8N;R) = Cl(8;R) x ...(N times tensor product)... x Cl(8;R)
I believe (but have not yet proven in detail) that you can similarly take the unions and do the completion to get what might be called a generalized real hyperfinite II_1, denoted here by hyperfinite II_1R.
The physical utility of the complex hyperfinite II_1 is that Cl(2;C) represents fermion creation/annihilation, and each little Cl(2;C) describes fermions in one little location.
My idea about the physical utility of hyerfinite II_1R is that each little Cl(8;R) describes Lagrangian physics in one little location, thus showing how Lagrangian stuff might fit inside algebraic stuff.
The local Lagrangian physics is based on my physics model that lets me calculate particle masses, force strengths, etc., as follows:
Cl(8;R) contains the basic structures used to formulate local Lagrangian physics:
Given those structures, it seems clear (at least to me) how they fit together to form a Lagrangian.
If you consider the 8-dim spacetime to only exist at high energies, and at low energies (where we do experiments) "freeze out" a preferred quaternionic submanifold that splits the 8-dim spacetime into a Kaluza-Klein type 4-dim physical spacetime and a 4-dim CP2 internal symmetry compact space, then (in a complicated but in my opinion realistic way) you end up with the Standard Model plus Higgs plus MacDowell-Mansouri gravity.
Philip Ball, in his book "The Devil's Doctor Paracelsus and the World of Renaissance Magic and Science" (Farrar, Straus, and Giroux 2006) said:
"... The sixteenth century has often been portrayed as a period of scientific as well as religious and political reformation. Traditionally, the scientific Luthers are Nicolaus Copernicus, who transformed astronomy, and Andreas Vesalius, who did the same for anatomy ... But there is another version of the story, and Paracelsus is at its center. ...Paracelsus lived from 1493 to 1541. ... He was not a part of the educated elite of sixteenth-century Europe but circled all his life on its fringes. ... "... My writings must not be judged by my language, but by my art and experience, which I offer to the whole world, and which I hope will be useful to the whole world." ...
in the philosophy of Paracelsus, science and rationalism do not compete with mysticism and superstition but blend with it, producing a ... wonderful and bizarre ... vision of the world ... It was this vision ... that defied the ... dogmatic interpretations of Classical ideas about the universe. ...
Paracelsus and his fellow iconoclast Cornelius Agrippa ... had ... the ... viewpoint that mankind had once known great things but had been corrupted in the era that separated legendary antiquity from their own day. ... think of Francis Bacon, of Robert Boyle, of Isaac Newton, with their visions of ... arcane knowledge ..
Magic was ... the precondition for science. ... for modern science to emerge ... Classical dogma had to give way to a form of empiricism that accepted the reality of ... unknowns ... such as the operation of occult forces. ...
much of contemporary science is itself occult in the Renaissance sense, insofar as it is "hidden" from our senses ... when ... Ernest Mach and Wilhelm Ostwald ... opposed the notion of atoms on the grounds that no one had ever seen one ... they were ... expressing suspicion of the occult ... modern science ... accommodated and formalized ... occult forces ... that seemed useful, such as magnetism and gravity ... Isaac Newton could not have formulated his gravitational theory without a belief in occult forces ...
For Paracelsus, all of nature was a form of alchemy. ... when Leonardo da Vinci speaks of geological water as the blood of the earth ... this was no metaphor ...
Paracelsus is the scientific positivist's worst nightmare. His work begins and ends in magic. Everything he writes is colored by his religious beliefs, which create a purposeful universe full of secret signs and symbols ... he wades in the numerological mire of the Kabbalah ...
Paracelsus says that he learned ... the doctrines of the Kabbalah ... in Constantinople. ... The word ... Kabbalah ... means "reception" in Hebrew, ... knowledge passed on only by word of mouth ... It was sacred wisdom that came directly from God. "Moses on the mount", said Pico ... protege ...[of]... Ficino (whom Paracelsus considered "the best of the Italian physicians") ... "received from God not only the Law, which he left to posterity written down in five books, but also a true and more occult explanation of the Law" ... the Kabbalah ... it revealed the how and the why of the world ...
Paracelsus was first of all a physician, and he ... sought to embed this "new" medicine within a comprehensive system of ... natural philosophy ... in 1533-34 ... Paracelsus visited the Tyrolean mines ...[and]... smelting works of the Fuggers ... and was struck by how the miners suffered from ailments peculiar to their profession ... Paracelsus ... petitioned the authorities for permission to practice as a physician. The response was predictable: "Because I did not appear in the garnishry of the doctors, I was dispatched with contempt ... The Burgomaster of Innsbruck had been used to doctors clad in silken robes at the court of princes, not in shabby rags grilled by the sun". ... the first manual of occupational health, On the Miner's Sickness and Other Miners' Diseases ... remained unpublished until ... 1567 ...
Paracelsus's cosmology ... did not and could not exclude theology ... his world was designed, and the Designer had left His mark everywhere on it. ... he firmly believed that things happen for a reason, that nature is mechanistic and follows rules, and that humankind could deduce and understand them. ...
Parcelsian matter is vitalized: it is an active substance, a living entity filled with creative potential ... all related to the Mysterium Magnum, the "one mother of all things", the "wonderful beginning" out of which came all of creation in a marvelous alchemical separation. "It is the greatest wonder of the philosophies", Paracelsus wrote, that "when the Mysterium Magnum is in its essence and divinity was full of the highest eternity, separatio started at the beginning of all creation". ...".
"... Paracelsus ... is said to have ridden a magical white horse ... and to have carried an enormous sword with magical powers ...".
Encyclopaedia Brittanica (2002 edition) said, about Paracelsus:
"... his father ... moved to Villach in southern Austria. There the boy attended the Bergschule, founded by the wealthy Fugger family of merchant bankers of Augsburg, where his father taught chemical theory and practice. Youngsters were trained at the Bergschule as overseers and analysts for mining operations in gold, tin, and mercury, as well as iron, alum, and copper-sulfate ores. The young Paracelsus learned from miners' talk of metals that "grow" in the earth, watched the seething transformations in the smelting vats, and ... gained insight ... that ... laid the foundations of his later remarkable discoveries in the field of chemotherapy.
In 1507, at the age of 14, he joined the many vagrant youths who swarmed across Europe in the late Middle Ages, seeking famous teachers at one university after another. During the next five years Paracelsus is said to have attended the universities of Basel, Tübingen, Vienna, Wittenberg, Leipzig, Heidelberg, and Cologne but was disappointed with them all.
He ... wondered how "the high colleges managed to produce so many high asses," ...
He was, however, delighted to find the medicine of Galen and the medieval Arab teachers criticized in the University of Ferrara, where, he ... was free to express his rejection of the prevailing view that the stars and planets controlled all the parts of the human body. ...
he set out upon many years of wandering through almost every country in Europe, including England, Ireland, and Scotland. ... Egypt, Arabia, the Holy Land, and ... Constantinople ...
After about 10 years of wandering, he ... 1527 ... had been appointed town physician and lecturer in medicine at the University of Basel ... he invited not only students but anyone and everyone. The authorities were scandalized and incensed by his open invitation. ... 1528, he ... had to flee ... toward Colmar in Upper Alsace ... Such ... travel for the next eight years allowed him to revise old manuscripts and to write new treatises. ...
In 1541 Paracelsus himself died in mysterious circumstances at the age of 48 at the White Horse Inn, Salzburg, where he had taken up an appointment under the prince-archbishop, Duke Ernst of Bavaria. ...".
According to math.QA/9906167 by Terry Gannon (who referred to the biography Evariste Galois (Birkhauser 1996) by L. Toti Rigatelli):
"... Evariste Galois was a brilliantly original French mathematician. Born shortly before Napoleon's ill-fated invasion of Russia, he died shortly before the ill-fated 1832 uprising in Paris. His last words: "Don't cry, I need all my courage to die at 20".Galois grew up in a time and place confused and excited by revolution. He was known to say "if I were only sure that a body would be enough to incite the people to revolt, I would offer mine". On May 2 1832, after frustration over failure in love and failure to convince the Paris math establishment of the depth of his ideas, he made his decision. A duel was arranged with a friend, but only his friend's gun would be loaded. Galois died the day after a bullet perforated his intestine. At his funeral it was discovered that a famous general had also just died, and the revolutionaries decided to use the general's death rather than Galois' as a pretext for an armed uprising. A few days later the streets of Paris were blocked by barricades, but not because of Galois' sacrifice: his death had been pointless ...".
In a review ( edited by Underwood Dudley ) of the biography Evariste Galois (Birkhauser 1996) by L. Toti Rigatelli in The American Mathematical Monthly for March 1998, Roger Cooke said:
"... In my mind's eye Galois and ...[the]... hero ... Marius Pontmorency ...[of]... Victor Hugo's Les Miserables ... became fused some years ago. ... Consider the parallels:Galois was born in 1811. According to Hugo, Marius' mother died in 1815 "leaving a child [Marius]."
Galois belonged to La Societe des Amis du Peuple. For Marius, Hugo invented a revolutionary society called Les Amis de l'A B C (a pun-read in French, it means The Friends of the Oppressed).
Galois plunged ... into a duel on May 30, 1832, apparently after losing the love of his Stephanie. Marius went to the barricades less than a week later, thinking he had lost his Cosette.
The badly wounded Galois was discovered by either a passing peasant or a soldier and taken to hospital. When Marius' reckless courage finally resulted in a wound that rendered him unconscious, his guardian angel Jean Valjean whisked him away and through the Parisian sewers to his grandfather's house, where he eventually recovered. ... Since Victor Hugo had a happy ending in mind, with Marius married to Cosette, it would not do to have the hero ... too badly wounded ... Unfortunately republicans and mathematicians, inferior to Victor Hugo in many respects, just as reality is inferior to fiction, neglected to provide for Galois. Our poor Evariste lacked a barricade; even worse, he lacked Jean Valjean, Victor Hugo's unbelievable amalgam of Arnold Schwarzenegger and St. Francis of Assisi, to snatch him up and return him to his family. He died on May 31. ...
the Russian translation of Andre Dalmas' Evariste Galois, revolutionnaire et geometre (Fasquelle, Paris, 1956), says that Galois was killed by one of his fellow republicans and that the cause of the duel was a woman suspected of being an agent of the police.
The Lexikon Bedeutender Mathematiker says he was lured into a duel, possibly orchestrated by the secret police.
The most obvious documentary evidence is Galois' letter to his fellow republicans, in which he asks pardon for those who killed him, saying that he is dying, "the victim of a cruel coquette and of two of her victims." ...
Dr. Rigatelli presents us with a different scenario, but one that pleases me, since it binds Galois even more closely to my friend Marius Pontmorency.
First of all she identifies the mysterious woman as Stephanie Poterin-Dumotel, daughter of the doctor who treated Galois during the latter part of his prison sentence. (This identification had been made earlier by Carlos Alberto Infantozzi, "Sur la mort d']Evariste Galois," Revue d'histoire des sciences 21 (1968), 157-160.)
Second, she prints some fragmentary letters whose originals, she says, were written in Galois' hand, but signed "Mademoiselle Stephanie D." ... Dr. Rigatelli believes he must have torn up the originals, then later regretted having done so and tried to reproduce them. ...
According to this scenario, Galois deliberately martyred himself in order to spark the insurrection. We are even given a report of a meeting of La Societe des Amis du Peuple, at which Galois presented his plan: he to be shot by one of their number, the others to spread the rumor that it was a police ambush in order to incite an insurrection, and the Society to be protected from the law by the letters portraying the affair as a duel. I was struck by the strong resemblance this scenario bears to the plot of Dostoevsky's The Possessed ...[in which]... the opportunistic revolutionary Verkhovensk tries to persuade Kirilov to time his suicide so as to bring maximal benefit to the cause. ...
... Galois mentions Victor Hugo in an obscure fragment, rather surprisingly, considering that Hugo was still young and not really famous in 1832, the author of a few volumes of poetry. He was also on the other side of the political fence from Galois at the time and was a peer of Louis Philippe's monarchy. As Louis Philippe moved rightward, Hugo moved leftward, causing him to write Les Miserables a generation later from a point of view closer to that of Galois. In the abovementioned fragment (given in the Russian translation of Dalmas' book) Galois writes: Here is Victor Hugo. The Renaissance, the Middle Ages, and last of all, me. Perhaps he is saying that Hugo portrays himself as the crowning achievement of human history. ...
we see Cauchy once again at his most unattractive, completely absorbed in his own thoughts and ignoring the brilliant work of a young mathematician who really needed his support. ...
none of the French mathematical establishment comes out of this biography looking good. Poisson and Lacroix delayed judging Galois' memoir on solvability of equations by radicals. When forced to render a judgment, they got it wrong, thereby depriving Galois of the credit he deserved and, as he later wrote, relegating him to the ranks of circle-squarers.
It isn't pleasant to read that the cranks are sometimes right, even in mathematics, but at least it isn't boring.
... the preface to Galois' memoirs, written while he was in prison ...
...(... The offense that landed Galois in prison in December 1830 was a toast to Louis Philippe at a banquet attended by many prominent people, among them Alexandre Dumas ... According to the official investigation, Galois claimed that he stood up, brandishing a knife, and shouted, "To Louis Philippe, if he betrays us!" ... others began to echo the toast, interpreting it as a direct threat to the King. At that point Alexandre Dumas left hastily through a window. ...)...... pointed out that he [Galois] was omitting the usual dedicatory page "crowded with the names and titles of some miserly prince...." Nor was he paying homage to any scientific patron. He wrote,
"I do not acknowledge anybody's advice or encouragement as being responsible for the good qualities of my work. I would be a liar if I did so. If I had a message for the great men of the world, . . . it would not be one of thanks." ...".