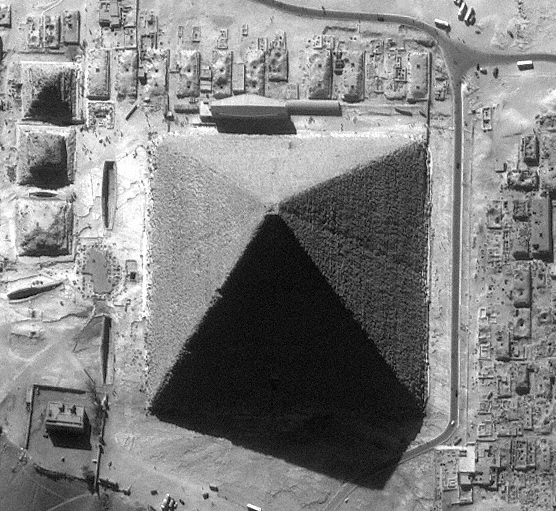
If the Great Golden Pyramid is a sound/electromagnetism transceiver, with the FACES of the Pyramid acting as large antennae, then THE GEOMETRY OF THE FACES of the Great Golden Pyramid should be designed to make then effective antennae. The faces of the Great Golden Pyramid were made up of the limestone casing stones, which unfortunately were removed by Egyptians for building material, so today we cannot see the Great Golden Pyramid as it was constructed. We now see only the rough stone core.
Jim Branson (knowhow@cyberhighway.net) says: Since the currently exposed outermost rough core stones are in fact rough (broken by impact versus cut or machined), the machined casing stones probably did not fit down against the rough stone (few points in contact). This makes the casing stones essentially a large homogeneous casing shell supporting itself in a cantilever arrangement. This has some interesting side effects, especially acoustic and electromagnetic. If the casing stones are a "thin shell", then the pyramid casing might act like a large organ tube (scaled from tubes about 12 inches in diameter and 3/16 inch thick). If the pyramid acted as one big tube, the appropriate thickness could be 6 feet thick. The Great Golden Pyramid might then be similar to a Big Bell, and the cantilever design would allow a fairly modest force to impart vibration to the casing bell shell.
Bells and other musical instruments are described in The Physics of Musical Instruments, by Neville Fletcher and Thomas Rossing, (Springer-Verlag 1991). The oldest bells it mentions are Chinese, between 1600 BC and 1100 BC. According to Joseph Needham (Science and Civilization in China, vol. IV:1, Cambridge 1962), in the time of about 1000 BC to 500 BC the Chinese Yo Chi listed four sources of sound: metal (bells); stone (flat and cylindrical (yi) ringing stones ), bamboo; and silk or leather.
What sort of connections to the pyramid interior should the receiver/transmitter Big Bell faces have? With respect to the receiver antennae, they should be connected to the receiving Mid Chamber. Both the North and South faces are so connected, by shafts that ran (but did not open into) the Mid Chamber, and ran (but did not open out on) toward the limestone outer faces of the Pyramid. That indicates to me that the Mid Chamber shafts ran between an intact inner plate (Mid Chamber Wall) and an intact outer plate (limestone casing and granite core wall). It will be very interesting to see what lies beyond the limestone stone blocking the South Shaft of the Mid Chamber, at the level of the floor of the Upper Chamber. It might possibly provide feed-back between Upper Chamber tranmsmissions and Mid Chamber receptions. With respect to the transmitter antennae, they should be connected to the transmitting Upper Chamber. Both the North and South faces are so connected. Both the North and South Upper Chamber shafts open into the Upper Chamber. The North Upper Chamber Shaft (pointing roughly toward the celestial North Pole) opened out through the North face. The South Upper Chamber Shaft would have so opened out through the South face, except that it was blocked at the time the Great Golden Pyramid was built by a gold-plated non-meteoritic iron plate about 0.5 RC by 0.5 RC, located a few meters from the outer surface of the pyramid. One "face" of the Pyramid about which I know very little is the top, or the 5th face, upon which transceiver-related material or apparatus could well have been placed. Due to my ignorance, I will not say much about it, but will mostly discuss the 4 larger faces.
If each of the 4 large faces of the Pyramid were simple isosceles triangles, then, even if their tops were slightly truncated, their antenna geometry would be simple: flat limestone casing over flat granite outer core. However, as we shall see, that is not the case. Neither the geometry of the granite outer core nor the geometry of the limestone casing is so simple, and the two geometries (outer core and casing) are different from each other. Since I don't know enough about how the geometries restict and modulate the signals, I will just mostly describe the geometries. However, I will cite here an interesting reference that deals with how geometries (of piano sounding boards, etc) affect signals (music): the book of Fletcher and Rossing, The Physics of Musical Instruments. In his 1973 book, Tompkins states: "Petrie found no evidence of hollowing along the lower-level casing stones, running along the base of the Pyramid, which have now been completely uncovered. A recent survey by two Italian scholars, Maragioglio and Rinaldi, indicates the casing stones ABOVE the base line may have been slightly sloped toward a central line." Since the casing base-line has no hollowing, each casing face cannot be divided into only 2 triangles, but can be divided into 3 triangles. My ideas about the geometry of the 3 triangles on each face of the Pyramid are a result of e-mail correspondence with Terry Nevin. Since each face of the Great Golden Pyramid is only an isosceles triangle, not equilateral, it is not possible for all 3 triangles on a given face to be equivalent. However, it is possible to make them equal in area, and that is what I do here. Here is a face-on side view of the Great Golden Pyramid showing 3 equal-area triangles per face:
Here is an edge-on side view of the Great Golden Pyramid showing 3 equal-area triangles per face:
Here is a top view of the Great Golden Pyramid showing 3 equal-area triangles per face:
The Great Golden Pyramid is shown as being truncated because it may never have had a conventional capstone. Some special object, like the eye above the pyramid on the US dollar bill, could have been located on the approximately 14.5 meter square top at course level 203, about 9.2 meters below the theoretical apex. Since the Great Golden Pyramid squares a circle, and is so associated with a hemi-sphere centered on the center of its base, you can ask at what "latitude" on the hemi-sphere is the intersection point of the 3 face triangles.
It is about 32.5 degrees, about 2.5 degrees north of the latitude of Giza, and the same angle as the north shaft of the Upper Chamber. Therefore, the intersection point of the 3 triangles of the north face point in the same direction as the north shaft of the Upper Chamber, approximately to the celestial north pole. This might support the ideas of Bauval and Hancock that the Great Golden Pyramid's associated hemisphere might be a representation of the Earth. The top platform would be a square of side somewhat less than 6 degrees, so that if represented the point on Earth with longitude of its location, Giza, and with latitude of 32.5 degrees, it would include Giza, at latitude 30 degrees, and also a region of the Eastern Mediterranean area roughly from Giza to Cyprus. The geography of the Giza Hemisphere
is roughly sketched above. The North Pole is beyond Leningrad from Giza. Cahokia is beyond London from Giza. Tokyo is beyond Xi'An from Giza. Andaman refers to the Andaman Islands beyond India. The Cape Verde Islands are beyond North Africa. The Brazil Basin is in the North Atlantic Ocean off South America. From South Africa, the East Africa Rift Valley goes north through the Highlands near the Nile Valley towards Giza, turning back southeast from the Red Sea down through the Indian Ocean to the SE Indian Ridge between Australia and Antarctica. As Bauval and Hancock have noted, the scale of the Giza Hemisphere is 1 to 43,200. Since 43,200 = 600 x 72, it may be related to the 4-dimensional version of the 3-dimensional icosahedron, the 600-cell whose construction involves the Golden Ratio, and the 72 root vectors of the E6 Lie algebra used in the D4-D5-E6-E7-E8 Vodou Physics model. The outer casing of finished limestone of the Great Golden Pyramid has long since been removed, leaving its core-masonry as what now appears as the outer faces. The core-masonry outer faces are NOT flat, but are hollowed. The dotted line in the figure above indicates the concavity of the casing hollowing, which would be hidden in the complete Pyramid, but is now visible:
The core-masonry of the Second and Third Pyramids are also exposed, but are clearly flat, not concave, so the concavity was not a building technique: it must have symbolized something. WHAT could be symbolized by the 3-triangle outer casing faces? Mathematically, the pattern looks like the Dynkin-Coxeter diagram of the D4 Lie algebra Spin(0,8) and like an octonion multiplication diagram. WHAT could be symbolized by the concave hollowed core-masonry base? One simple representation is that, in units of Pyramid Inches, the total outer perimeter of the Great Golden Pyramid at its base, following the indentations, is 36,525.6 PI; but going straight from corner to corner it is 36,524.2 PI. The indented path represents the sidereal year of 365.256 days, while the straight path represents the mean solar tropical year of 365.242 days. On a more abstract level, the plan of the Giza plateau, including the 3 Pyramids and their 2 vanishing points, was constructed based on the elliptic spherical geometry connecting structures on a 2-dimensional Earth surface with stars on a 2-dimensional celestial sphere. The base of 4 concave faces could represent hyperbolic geometry
as the red region in the center of the hyperbolic Poincare disc. In hyperbolic geometry, as in Minkowski spacetime, time and space are represented differently. That is not the case in elliptic spherical geometry, in which space and time are indistinguishable. This can be expressed by saying that the elliptic spherical metric has signature ++... while the hyperbolic metric has signature -+... (where ... indicates all the spatial dimensions have + signature). Consider the case of 2 dimensions, 1 time and 1 space. Elliptical spherical geometry is the geometry of circles on a sphere, and all the circles look alike. Hyperbolic geometry is the geometry of a disc whose boundary is a circle. The disc is made up of two different kinds of things:
_______
The timelike things are arcs of circles
that are perpendicular to the boundary circle.
4 of these form the boundary of the red region
in the figure above (by Juha Haataja at CSC).
In higher dimensional spacetime,
these arcs would still be 1-dimensional arcs.
The spacelike things are circles inside the disc that
are tangent to the disc at its boundary circle.
They are called horocycles. In higher dimensional spacetime,
the horocycles would be spheres instead of circles.
If you look only at a spacelike horocycle in
the hyperbolic spacetime, you see that,
since it is a sphere, it has spherical geometry.
In N-dimensional hyperbolic geometry,
spacetime is divided into 1-dimensional time
and
spherical (N-1)-dimensional space.
If I represents an interval of real numbers
(such as zero to (but not including) pi)
and if S3 is the 3-sphere and S7 is the 7-sphere,
then I x S3 or I x S7
could be a hyperbolic geometric representation
of the spacetimes of the D4-D5-E6-E7-E8 VoDou Physics model.
As Euclidean geometry is intermediate between
elliptic spherical geometry and hyperbolic geometry,
the Pyramids at Giza show all 3 types of geometry:
The plan of the 3 pyramids on the Giza plateau,
on the surface of the spherical Earth, has SPHERICAL GEOMETRY;
Each pyramid itself is a 4-sided pyramid, and has EUCLIDEAN GEOMETRY.
If there were a mirror image pyramid below the physical pyramid
that is above ground, they would not form an octahedron
because the faces are not equilateral triangles. They would
form a 4-sided bypyramid, whose symmetry group is the D4
finite group corresponding by the McKay correspondence to Spin(8).
The horizontal cross-sections of the core-masonry
of the Great Pyramid has HYPERBOLIC GEOMETRY.
If the Great Pyramid is regarded as a bunch of
horizontal hyperbolic planes stacked up vertically,
or H2 x I where H2 is the hyperbolic plane and I is an interval,
then its resulting geometry is an example of a
3-dimensional geometry that is homogeneous but not isotropic.
Any horizontal section of such a Great Pyramid would
have hyperbolic geometry, but
any vertical cross-section
(such as the plane of its chambers and shafts)
would have Euclidean geometry.
Spherical, Euclidean, and Hyperbolic geometries are all
subgeometries of LIE SPHERE GEOMETRIES.
Lie sphere geometries are the geometries of hyperspheres
embedded in Spherical space, Euclidean space, or Hyperbolic Space.
As homogeneous spaces, ordinary spheres Sn are
Spin(n+1) / Spin(n)xSpin(1)
where Spin(1) is the 2-element group {-1, +1},
and
Lie spheres are Spin(n+2) / Spin(n)xSpin(2)
where Spin(2) = U(1).
Since it uses such structures as Spin(10) / Spin(8)xU(1)
the D4-D5-E6-E7-E8 Vodou Physics model
can be said to be based on Lie sphere geometry.
If you have read all this, you have probably noticed that
I have not said much about the content of the messages
sent and received by the Pyramid transceiver,
except that it is based on sound, and therefore music,
which is closely related to mathematics.
Also, not much is said about with whom
the Pyramid civilization would be communicating.
I like to think that the Great Golden Pyramid may be
a transceiver for communication among higher civilizations
of an InterGalactic Internet,
or even higher civilizations who can move among the Many-Worlds.
The conjecture that the Great Golden Pyramid is a transceiver
is based on e-mail conversation with J. C. Paul -
- however I am solely responsible for errors of fact that may appear.
More interesting math (and language) stuff
is on an Egyptian Hieroglyphics page.
Some comments on human civilization
at the time of the building of the Great Golden Pyramid
are HERE.
REFERENCES:
Many of the facts and images are taken
from the following references - (however,
I am responsible for any erroneous speculations):
Bauval, R., The Orion Mystery (Crown, 1994, 1995);
Bauval, R. and Hancock, G., The Message of the Sphinx
(Crown, 1996);
Berry, L., and Mason, B., Mineralogy (W. H. Freeman, 1959);
Cecil, T., Lie Sphere Geometry (Springer-Verlag, 1992);
Fletcher, N., and Rossing, T., The Physics of Musical
Instruments (Springer-Verlag, 1991);
Hancock, G., Fingerprints of the Gods (Crown, 1995);
Helgason, S., Groups and Geometric Analysis (Academic 1984);
Huang, K., and Huang, R., I Ching (Workman, 1987);
Kappraff, J., Connections (McGraw-Hill, 1991);
Kaufmann, W., Universe (Freeman, 1988, 1991, 1994);
Lemesurier, P., The Great Pyramid Decoded (Element, 1977, 1993);
Lemesurier, P., The Great Pyramid, Your Personal Guide
(Element, 1987);
Levy, S., Automatic Generation of Hyperbolic Tilings,
in The Visual Mind, Emmer, ed. (Leonardo 1993);
Montesinos, J., Classical Tessellations and Three-Manifolds
(Springer-Verlag 1987);
Tompkins, P., Secrets of the Great Pyramid (Allen Lane 1973);
Weeks, J., The Shape of Space (Marcel Dekker 1985).
For doing calculations about resonant frequencies of the structures of the Great Golden Pyramid, Mike Mitton has pointed out that the CSG web site has a very useful Calculators and Converters web page.
......