

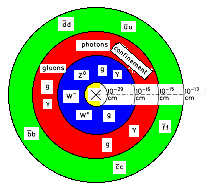
This view of the GUT monopole raises the possibility that it may catalyze the decay of the proton ...". ]...SU(5) GUT Monopole formation ends and the Inflationary X-Boson Higgs mechanism eliminates the relic Monopoles.
- According to The Early Universe, by Kolb and Turner (1994 paperback edition, Adddison-Wesley, page 526): "... the full symmetry of the GUT cannot be manifest; if it were the proton would decay in 10^(-24) sec. The gauge group ... must be spontaneously broken to [ SU(3) x SU(2) x U(1) ]. For SU(5), this is accomplished by ... masses of the order of the unification scale for the twelve X ... gauge bosons. ...[
X color charges X electric charges 3 3 3 X X red red -4/3 -1/3 3 3 3 X X green green -4/3 -1/3 3 3 3 X X blue blue -4/3 -1/3 X X X 2 2 antired antigreen antiblue +4/3 +4/3 +4/3 X X X 2 2 antired antigreen antiblue +1/3 +1/3 +1/3]... Thus, ... at energies below 10^14 GeV or so the processes mediated by X ... boson exchange can be treated as a four-fermion interaction with strength ... [proportional to 1 / M^2 ] ... where M = 3 x 10^14 GeV is the unification scale. ... these new ... interactions are extremely weak at energies below 10^14 GeV. ... the proton lifetime must be ...[about]... 10^31 yr. ...".
- In The Early Universe (paperback edition Addison-Wesley 1994) Kolb and Turner say (at p. 526): "... SU(5) GUT ...[has]... at the very least one complex 5-dimensional Higgs. The 5-dimensional Higgs contains
- the usual doublet Higgs required for W-Boson SSB ...[which]... must acquire a mass of order of a few 100 GeV and
- a color triplet Higgs ... which can also mediate B,L [baryon,lepton] violation. The triplet component must acquire a mass comparable to ... M = 3 x 10^14 GeV ... to guarantee the proton's longevity, ...".
3 3 3
3 3 3
3 3 3
2 2
2 2and then to the Standard Model SU(3) x SU(2) x U(1) with the usual Higgs doublet with VEV around 250 GeV.
After the Inflation Era, Our Universe begins its current phase of expansion

controlled by Gravity according to a MacDowell-Mansouri Mechanism based on the Conformal Group Spin(2,4) = SU(2,2) with 15 generators:
According to gr-qc/9809061 by R. Aldrovandi and J. G. Peireira: "... By the process of Inonu-Wigner group contraction taking the limit R -> 0, ...[where R is the de Sitter pseudo-radius, the] ... de Sitter group... [ whether of metric ... (-1,+1,+1,+1,-1) or (-1,+1,+1,+1,+1) , is]... contracted to the group Q, formed by a semi-direct product between Lorentz and special conformal transformation groups, and ... de Sitter space...[is]... reduced to the cone-space N, which is a space with vanishing Riemann and Ricci curvature tensors. As the scalar curvature of the de Sitter space goes to infinity in this limit, we can say that N is a spacetime gravitationally related to an infinite cosmological constant.".
If the 2+4 = 6-dimensional spacetime on which the full Conformal Group Spin(2,4) acts linearly is viewed in terms of an elastic Aether, its rigidity would correspond to the VEV of the X-Boson Higgs Condensate on the order of 10^14 GeV. Since the action of the Conformal Group Spin(2,4) = SU(2,2) is nonlinear on 4-dimensional physical spacetime, the 4-dimensional elastic Aether can, within the Conformal Expanding Domain of Our Universe, be deformed by Special Conformal and Dilation transformations without the restrictions of X-Higgs VEV rigidity on the order of 10^14 GeV.
The Aldrovandi-Peireira paper shows that
What about the other Generators?
The 1 Scalar Dilation Generator corresponds to the Scalar Higgs of the W-Bosons, with VEV 250 GeV, that gives mass to Ordinary Matter in Our Universe.
Those 15 Conformal Group Spin(2,4) = SU(2,2) Generators indicate that the basic tree-level ratio Dark Energy : Dark Matter : Ordinary Matter is 10 : 4 : 1 = 67 : 27 : 6 . After taking into account the history of Our Universe to the Present Time, that ratio is calculated to be, as of Now, consistent with observations including WMAP:
After conventional expansion of our universe begins, some regions of our Universe become Gravitationally Bound Domains (such as, for example, Galaxies) in which the 4 Conformal GraviPhoton generators are frozen out, forming domains within our Universe like IceBergs in an Ocean of Water. Within each Gravitationally Bound Domain, spacetime (regarded as Aether) is incompressible with a rigidity on the order of the W-Boson Higgs VEV = 250 GeV.
On a large scale (billions of light years), the Gravitationally Bound Domains are roughly traced out by Galaxies and Clusters of Galaxies

so the the white dots would be the Gravitationally Bound Domains (like rigid pennies on an expanding balloon, or rigid raisins in an expanding cake) and the black background would be the Conformal Expanding Domain of Our Universe.
When the Gravitationally Bound Domains begin to form as Galaxy Cluster Structures in the early stages of the current phase of expansion of Our Universe, according to a 6 December 2006 caption to ESO PR Photo 45/06
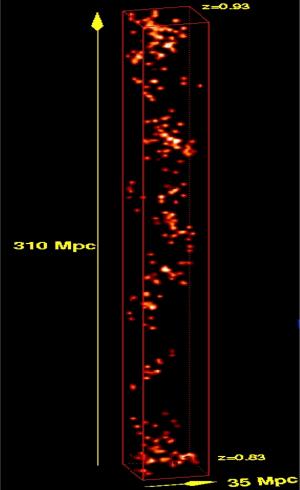
"... Spatial, three-dimensional distribution of galaxies in a slice of the Universe as it was 7 billion years ago, based on the VVDS study: brighter areas represent the regions of the Universe with most galaxies. Astonishingly, the galaxy distribution - the 'building blocks' of the large scale structure - takes the shape of a helix at this primordial epoch. ...". Such a helical structure suggests that helical magnetic fields might be involved in galaxy formation. Further, Battaner et al, in in astro-ph/9801276, astro-ph/9802009, and astro-ph/9911423, suggest that the simplest network pattern for distribution of superclusters of galaxies that is compatible with magnetic field constraints
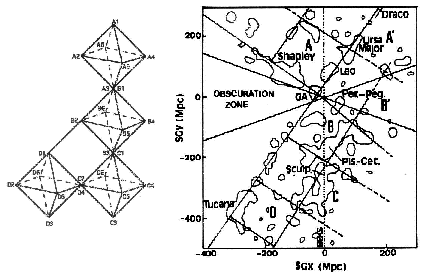
is made up of octahedra contacting at their vertexes, which is related to a tiling of 3-dim space by cuboctahedra and octahedra, and also to the heptaverton of Arthur Young and octonionic structures of Onar Aam.
Within each Gravitationally Bound Domains there can exist Islands of Conformal Expansion in which all 15 generators of Conformal Spin(2,4) = SU(2,2) remain effective,

like Puddles of Water (red) on an IceBerg (blue) floating in an Ocean of Water (red), so the overall structure of Our Universe in terms of Gravitationally Bound Domains (pennies, raisins, IceBergs) and Conformal Expanding Domains (balloon, cake, water) is quite complicated.
To get some feeling for this structure, begin by considering Clusters of Galaxies to be the largest Gravitationally Bound Domains and then looking at the next level down in sixe, Galaxies. As Hartmann and Miller say in their book Cycles of Fire (Workman Publishing 1987)
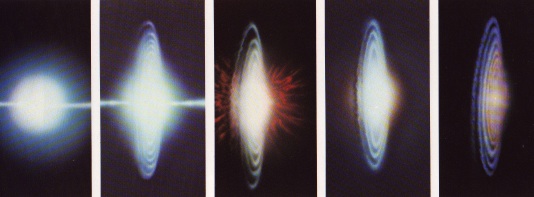
Going down one more level in size, to Stars and Stellar Systems like Our Solar System, Hartmann and Miller describe
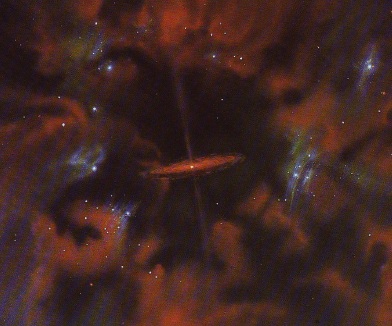
"... a star just formed ...[in]... its disk-shaped cocoon nebula some of which is being blown out in bipolar jets ... near a dark molecular cloud ... embedded in a ... nebular region ... The dust in the cocoon reddens the star's light ...".
Kohji Tomisaka of Niigata University says in astro-ph/9911166: "... the star formation process ... angular momentum transfer in the contraction of a rotating magnetized cloud is studied with axisymmetric MHD simulations. Owing to the large dynamic range covered by the nested-grid method, the structure of the cloud in the range from 10 AU to 0.1 pc is explored. First, the cloud experiences a run-away collapse, and a disk forms perpendicularly to the magnetic field, in which the central density increases greatly in a finite time-scale. In this phase, the specific angular momentum j of the disk decreases to about 1/3 of the initial cloud. After the central density of the disk exceeds about 10^10 cm ^(-3) , the infall on to the central object develops. In this accretion stage, the rotation motion and thus the toroidal magnetic field drive the outflow. The angular momentum of the central object is transferred efficiently by the out\flow as well as the effect of the magnetic stress. ... the seeding region (origin of the outflow) ... expands radially outward. This outflow is driven by the gradient of the magnetic pressure of the toroidal magnetic fields ... which are made by the rotation motion ... The magnetic fields exert torque on the outflowing gas to increase its angular momentum. On the other hand, they exert torque on the disk to decrease the angular momentum ... [in about 7000 years] ... the outflow expands and reaches ... [about 4 AU] ... The angular momentum distribution at that time ... has been reduced to ... a factor of 10 ^(-4) from the initial value (i.e. from 10^20 cm^2 s ^(-1) to 10^16 cm^2 s ^(-1)). ... the coupling between gas and magnetic fields ... becomes stronger as long as we consider the seeding region, indicating that the mechanism of angular momentum transfer works also in the later stage of the evolution [after 7000 years]. ...".
If you look closely at the central star in the star-formation image above, you might see Birkeland Current Loops (image from thesurfaceofthesun.com web page) that look up close like Solar Coronal Loops (image from electric-cosmos.org/sun.htm web page).

Up close, Birkeland Current Loops are seen to have braided filament structure (Cygnus Loop image from antwrp.gsfc.nasa.gov.

The scale of Birkeland Current Loops extends beyond Stellar to Galactic (images, SOHO of Sun and NRAO of Fornax A from thunderbolts.info webpage, which said as to NGC "... a tiny but energy-dense plasmoid at the center of the galaxy ... Fornax A ... discharges energy along oppositely-directed Birkeland filaments (invisible in this image) into the radio lobes. Diffuse currents loop back from the lobes to the spiral arms, where their increasing density triggers star formation as they return to the central plasmoid. ..." ...).

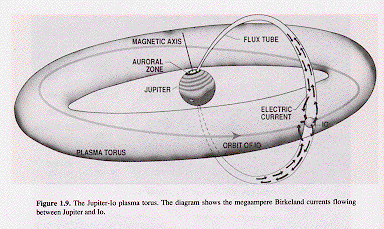
The scale also extends down to Planetary, as is seen in the Jupiter-Io system (image from Anthony Peratt's book Physics of the Plasma Universe (Springer-Verlag 1992)).
The scale may also extend down to Asteroidal. According to a 17 September 1994 article by Jeff Hecht in the New Scientist: "... inclusions ... in chondrules ... in chondrites, the commonest meteorites ...[were]... heated ... to about 2000 kelvin at the birth of the solar system, 4.6 billion years ago. ...[possibly by]... Lightning ... and ... magnetic discharges ... laser tests ... to model the intense visible and infrared light expected near electric or magnetic discharges ... produced dark structures ... remarkably similar to .... inclusions found in chondrules ...".
As can be seen from the image below (adapted from some of the above images and also An Introduction to Modern Astrophysics, by Carroll and Osterlie (Addison-Wesley 1996), Solar System Evolution, by Stuart Ross Taylor (Cambridge 1992), and B. V. Vasiliev's papers astro-ph/0002048 and astro-ph/0002171), Angular Momentum, Magnetic Dipole Moment, and Mass are systematically related or Stars and Stellar Systems and their components.
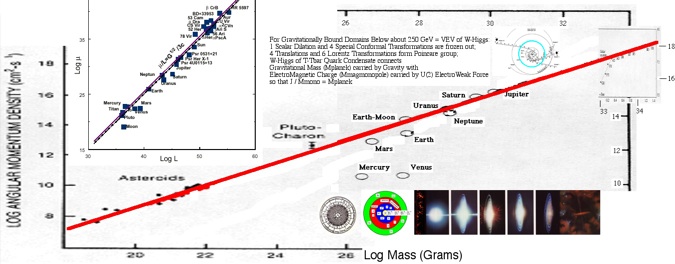
Angular Momentum J and Magnetic Dipole Moment P are related by a constant that is on the order of unity ( J = P ) (natural units) due to Gravity-Induced Electric Polarization of matter.
As to the relationship between Angular Momentum J and Mass (which, due to the Angular Momentum - Magnetic Dipole Moment relationship, implies a relationship between Magnetic Dipole Moment and Mass), Jack Sarfatti's paper wessonI.PDF describes a 1981 paper by Paul Wesson in which Wesson plotted total angular momentum J against mass M for the solar system, double stars, star clusters, spriral galaxies, the Coma cluster, and the local supercluster in which Wesson found that Angular Momentum J and Mass M are related by a constant p such that
J/M = p M.
Wesson's observations indicate approximately, that
p = 1 / alpha_EM = 137 (natural units G = hbar = c = 1).
For Elementary Specific Angular Momentum J/M = hbar, in natural units where hbar = 1 and the unit of mass is the Planck mass Mplanck:
M = Mplanck / 137
which is roughly the mass of an SU(5) Magnetic Monopole.
Wesson's observations are consistent with a Compton Radius Vortes Kerr-Newman Black Hole related to the Wesson Force. The equation (in units with G = c = hbar = 1) for a Kerr-Newman Black Hole with coincident outer and inner event horizons and with Q = 1
meaning that the Black Hole Core has UNIT amplitude to absorb or emit a gauge boson, in accord with Feynman's statement in his book QED (Princeton 1988): "... e - the amplitude for a real electron to emit or absorb a real photon. It is a simple number that has been experimentally determined to be close to -0.0854... the inverse of its square: about 137.03... has been a mystery ... all good theoretical physicists put this number up on their wall ..."
is Q^2 + (J/M)^2 = 1 + (J/M)^2 = M^2. Dividing through by M^2, you get
For the Wesson force for which J = p_wesson M^2 with p_wesson = 1 / alpha_EM
so that 1 - (1/M)^2 = 137^2 and 1/M = sqrt(1 -137^2) = 137 i = 137 exp(pi/2)
Then the magnitude | 1 / Mwesson | = 137 which (since the units are natural units with G = c = hbar = 1) implies that
which is consistent with Wesson's observation that
The Linear Angular Momentum, Magnetic Dipole Moment, and Mass Relationships hold in Gravitationally Bound Domains, which are characterized by
If the 1+3 = 4-dimensional spacetime on which the 6+4 = 10-dimensional Poincare Group Spin(1,3) + 4-Translations acts linearly is viewed in terms of an elastic Aether, its rigidity would correspond to the VEV of the W-Boson Higgs Condensate on the order of 250 GeV. Within Gravitationally Bound Domains, since Special Conformal and Dilation transformations are frozen out, the rigidity of the 4-dimensional elastic Aether corresponds to the W-Higgs VEV of about 250 GeV.
Although the Wesson angular momentum / mass relationship covers a very wide range of mass scales (at least from Asteroids to Stars and Stellar Systems), it is not Universal. Some other angular momentum / mass relationships are:
The differences may be that the Wesson relationship involves a combination of ElectroMagnetic and Gravity forces during Collapse/Formation, while, for the others, the forces involved are:
Can a laboratory-scale experiment extend the Wesson-type relationship between Angular Momentum J and Magnetic Dipole Moment P to sub-asteroid laboratory mass scales ?
Saul-Paul Sirag, in his 3 November 2000 paper Vigier III: "Gravitational Magnetism: an Update", says:"... The most straightforward test ... would be to measure directly the magnetic field of a rotating neutral body (which is not also a ferromagnetic substance). Blackett ... suggested that a 1-meter bronze sphere spun at 100 Hz would do nicely, except that this is the maximum safe speed, and there are severe problems in nulling out extraneous magnetic fields. With modern SQUIDs and mu-metal shielded rooms, such an experiment can be attempted. Exactly such an experimental design ... was described at the SQUID '85 conference in Berlin. However, the results of this experiment have not been published. ...".
The MicroScale Particle Physics proportionality between Q and M obviously does not extend far into the MacroScale, since Asteroids, Planets, and Stars do not have large net Electric Charges.
The Kerr-Newman Black Hole structure of a Compton Radius Vortex has the property that the square of the electric charge Q plays the same role as the square of J/M (specific angular momentum, or angular momentum over mass) in that their sum, relative to the square of the mass, determines whether the outer and inner event horizons are
Jack Sarfatti relates Compton Radius Vortex structure of Elementary Particles to the formula of Saul-Paul Sirag (based on earlier work of Blackett and Schuster, and perhaps Pauli) in his 1979 Nature paper Gravitational Magnetism (vol. 278 pp. 535-538, 5 April 1979), in which Saul-Paul Sirag says: "The gravi-magnetic hypothesis proposes that a rotating mass, measured in gravitational units, has the same magnetic effect as that of a rotating charge, measured in electrical units. The respective force constants determine this relationship
where G is the gravitational constant, M is mass, k is the Coulomb constant, and Q is electric charge. ... Thus the ratio of magnetic moment P to angular momentum J for a sphere of mass M, density factor f, radius r, angular velocity w, and magnetic field B is (in SI units [with magnetic permeability muo of the vacuum]):
... A priori, we should expect a correlation between P and J. ... The surprise is that this correlation ratio, P/J, should turn out to be close to P = ( G^(1/2) /2 k^(1/2) ) J. ... The gravi-magnetic hypothesis (stated in [ Log = log_10] form) predicts a P/J of -10.37. The mean P/J of the data points plotted in Fig. 1 is -11.13 with a standard deviation of 0.42. ... Therefore, for a given value of the angular momentum J, the gravi-magnetic hypothesis overstates the magnetic dipole moment P by a factor of 10^(-10.37 - (-11.13)) = 10^0.76 = 5.75. Saul-Paul Sirag says: "... the deviation from the gravi-magnetic hypothesis line is fairly systematic. ... deviations ... may well be due to electrical-magnetic effects. ... [ P / J = G^(1/2) / 2 k^(1/2) ] predicts a surface field about three times greater than that measured at the surface of the Earth. ... the Earth is not a uniformly dense sphere ... At the Earth's surface ... [ ( (5/4) 4 pi B / muo ) ( r / f w M ) = G^(1/2) / 2 k^(1/2) ] predicts a B of 2.1 x 10^(-4) T. That is not, however, a great deal more than the 1.4 x 10^(-4) T that [the equation] predicts for the surface of the Earth's core. ... this core magnetism predicted by the gravi-magnetic equation is greater than the magnetic field of 6 x 10^(-5) T measured at the Earth's surface. ... This is what we expect if we suppose that gravitational magnetism is modified by an electrical-magnetic effect stronger at the Earth's surface than in the interior. ...".
B. G. Sidharth, in physics/9908004, says: "... We first observe that as is known an assembly of Fermions below the Fermi temperature occupies each and every single particle level, and this explains the fact that it behaves like a distribution of Bosonic phonons: The Fermions do not enjoy their normal degrees of freedom. ... [there is a] Bosonization or semionic effect. ... Let us now consider an assembly of N electrons. As is known, if N+ is the average number of particles with spin up, the magnetisation per unit volume is given by
M = mu ( 2 N+ - N ) / V
where mu is the electron magnetic moment. At low temperatures, in the usual theory, N+ = N / 2, so that the magnetisation ... is very small.
On the other hand, for Bose-Einstein statistics we would have, N+ = N. With the above semionic statistics we have,
If N is very large, this makes an enormous difference ... Let us use ... the case of Neutron stars. In this case, as is well known, we have an assembly of degenerate electrons at temperatures about 10^7 K, whereas the Fermi temperature is about 10^11 K ... So the above considerations apply. In the case of a Neutron star we know that the number density of the degenerate electrons, n = 10^31 per c.c. So ... remembering that mu = 10^(-20) G (Gauss), the magnetic field near the Pulsar is about 10^11 G < 10^8 Tesla, as required. Some White Dwarfs also have magnetic fields. If the White Dwarf has an interior of the dimensions of a Neutron star, with a similar magnetic field, then remembering that the radius of a White Dwarf is about 10^3 times that of a Neutron star, its magnetic field would be 10^(-6) times that of the neutron star, which is known to be the case. It is quite remarkable that the above mechanism can also explain the magnetism of the earth. As is known the earth has a solid core of radius of about 1200 kilometers and temperature about 6000 K. This core is made up almost entirely of Iron (90%) and Nickel (10%). It can easily be calculated that the number of particles N = 10^48 , and that the Fermi temperature is about 10^5 K. In this case we can easily verify ... that the magnetic field near the earth's surface is about 1 G, which is indeed the case. It may be mentioned that the anomalous Bosonic behaviour ... would imply a sensitivity to external magnetic influences which could lead to effects like magnetic flips or reversals. ... Remembering that the core density of Jupiter is of the same order as that of the earth, while the core volume is about 10^4 times that of the earth, we have in this case, N = 10^52 , so that the magnetization ... is about 10^4 times the earth's magnetism, as required. ....".
According to a 23 March 2006 ESA news web page: "... Martin Tajmar, ARC Seibersdorf Research GmbH, Austria; Clovis de Matos, ESA-HQ, Paris; and colleagues have measured ... a gravitomagnetic field ... generate[d] ...[by]... a moving mass ... Their experiment involves a ring of superconducting material rotating up to 6 500 times a minute. Superconductors are special materials that lose all electrical resistance at a certain temperature. Spinning superconductors produce a weak magnetic field, the so-called London moment. The new experiment tests a conjecture by Tajmar and de Matos that explains the difference between high-precision mass measurements of Cooper-pairs (the current carriers in superconductors) and their prediction via quantum theory. They have discovered that this anomaly could be explained by the appearance of a gravitomagnetic field in the spinning superconductor (This effect has been named the Gravitomagnetic London Moment by analogy with its magnetic counterpart). ... Although just 100 millionths of the acceleration due to the Earth's gravitational field, ...[ gr-qc/0603033 says "... the peaks ... "only" 100 micro g ... are 30 orders of magnitude higher than what general relativity predicts classically ..."]... The electromagnetic properties of superconductors are explained in quantum theory by assuming that force-carrying particles, known as photons, gain mass. By allowing force-carrying gravitational particles, known as the gravitons, to become heavier, they found that the unexpectedly large gravitomagnetic force could be modelled. ... The papers can be accessed on-line at the Los Alamos pre-print server using the references: gr-qc/0603033 and gr-qc/0603032. ...".
...