The Tarot is a well-known mystical structure that may have its origin in ancient cultures. This page is a view of the Tarot from the point of view of underlying mathematical structures, to see whether it might represent well-defined mathematical objects that can be used to build models such as the D4-D5-E6 physics model that describe physical phenomena in ways that are consistent with experimental observations. If so, it may indicate that the useful mathematical objects may be some sort of archetypes built into human brains, transmitted through the centuries embedded in games (as the Tarot). Other things that might similarly represent archetypal structures include, but are not limited to: the I Ching, which models binary numbers and 8x8 Clifford algebras; and the Torah and the Sefirot of Kabbala.
The TORAH is a sequence of 304,805 letters.
The SEFIROT is a pattern of 10 vertices linked by 22 lines.
Why does the Sefirot use only 22 lines ?
Symmetry of the 22 Hebrew letters plus 5 Finals.
3x3x3 Magic Cube with 27 elements.
3^5 = 243 was used by Plato to construct a musical scale.
To describe them, use the notation 0 = Fool; 1, ... , 21 = major arcana; K = King, Q = Queen, k = knight or prince, j = jack or princess; and suits of the minor arcana being s = swords, w = wands, c = cups, p = pentacles or disks. The 22 Major Arcana cards (including 0) can be identified with the 22-letter Hebrew alphabet, in which 3 letters are Elementary (compare quaternion imaginaries); 7 letters are Double (compare octonion imaginaries); and 12 letters are Simple (compare signs of the zodiac). The total number of Minor Arcana cards is 4(4+10) = 56 If the 4 knight or prince cards are omitted, and the 4 princess cards are termed jacks, the remainder of the Minor Arcana is just the commonplace 52-card deck of playing cards. All 78 Tarot cards can be identified with the 78 dimensions of the E6 Lie algebra of the D4-D5-E6 physics model.
First, try to visualize 6-simensional space this way:
Start with the 2-dimensional space of the Gaussian integers
of the complex numbers, which have as a basis +1, +i, -1, and -i
+i
|
-1 -- 0 -- +1
|
-i
You can extend this pattern to cover
the entire 2-dimensional complex number plane with a square lattice.
Notice that the origin 0 (zero),
as well as any other vertex point in the square tiling of the plane,
has 4 nearest neighbor points (in the case of 0, the nearest
neighbors are the 4 points shown: +1, +i, -1, and -i ).
Now consider each of those 4 nearest neighbor points to be
"doorways" to one of 4 "new" dimensions "beyond" the 2-dim plane.
That gives you 2 + 4 = 6 dimensions in total,
and it is one way to visualize 6-dimensional space.
Now look at the origin in 6-dim space,
and ask:
What are the "nicely-symmetrical" ways we can arrange nearest
neighbor points to get a lattice that "tiles" 6-dim space?
The most dense 6-dim lattice that is known is the E6 lattice,
in which each point has 72 nearest neighbors.
If you combine the 6 "old" dimensions of 6-dim space
with the 72 "new doorway" dimensions of the nearest neighbors,
you get the 6 + 72 = 78 dimensions that correspond to
the 78-dimensional E6 Lie algebra
that I use in the D4-D5-E6 physics model,
and
that also (in my opinion) correspond to the 78 cards of the Tarot.
--------
One (of many) interesting things about the 78-card Tarot is
that the popular 52-card Playing Card Deck is a subset of it,
and
there is a corresponding 52-dimensional subalgebra, called F4,
of the 78-dimensional Lie algebra E6.
Just as I started out with 52 Playing Cards
before I learned about Tarot,
when I started building my physics model
I tried to use the Lie algebra F4
before I realized that I needed E6.
F4 was smaller and less complicated,
so when I started with it
I could build a sort of "approximate" model.
Then later,
when I realized that F4 was not big enough,
it was natural for me to go beyond 52-dim F4
to 78-dim E6 and the D4-D5-E6 physics model.
(You can visualize 52-dim F4 by starting with a 4-dim space structure
in which each point has 48 nearest neighbors,
for 4 "old" dimensions plus 48 "new dooorway" dimensions,
to get 4 + 48 = 52 dimensions of F4.)
---------
To see the Tarot structure of the D4-D5-E6 physics model,
start with the major arcana card 1,
and add cards as follows: [ For another version click HERE. ]
1 This is
Spin(2) = U(1)
-----------------------------------------------------------------
2 3
1 This is
Spin(3) = SU(2) = Sp(1) = S3
-----------------------------------------------------------------
2 3
1 6 This hexagon is
Spin(4) = Spin(3)xSpin(3)
4 5 = SU(2)xSU(2)
= Sp(1)xSp(1)
= S3 x S3
-----------------------------------------------------------------
7 8 9
2 3 10
1 6 This is Spin(5) = Sp(2).
4 5
-----------------------------------------------------------------
7 8 9
2 3 10
1 6 15 This nest of 2 hexagons
is Spin(6) = SU(4).
4 5 14
11 12 13
-----------------------------------------------------------------
16 17 18 19
7 8 9 20
2 3 10 21
1 6 15 This is Spin(7).
4 5 14
11 12 13
---------------------------------------------------------------------
16 17 18 19
7 8 9 20
2 3 10 21 This is D4=Spin(8).
Here I have used all
1 6 15 Qc four of the 9-cards
and three of the 10-cards.
4 5 14 Qw Now there are
3 nested hexagons.
11 12 13 Qs Compare the 28 Hsiu.
Ks Kw Kc Kp
-------------------------------------------------------------------
Qp ks kw kc kp
16 17 18 19 js
7 8 9 20 jw This is Spin(9).
2 3 10 21 jc
1 6 15 Qc
4 5 14 Qw
11 12 13 Qs
Ks Kw Kc Kp
---------------------------------------------------------------------
Qp ks kw kc kp
16 17 18 19 js
7 8 9 20 jw
2 3 10 21 jc This is D5=Spin(10)
with dimension 45 = 28+16+1.
1 6 15 Qc 0 The 16 form an 8-dim complex space
with 8-dim real Shilov boundary.
4 5 14 Qw 9c Compare the 8 Immortals.
11 12 13 Qs 9w Here there are
4 nested hexagons.
Ks Kw Kc Kp 9s
jp 10s 10w 10c 10p
---------------------------------------------------------------------
To get to 78-dim E6 from 45-dim D5=Spin(10), you need 33 more cards:
32 = 4x8 from the minor arcana plus one (for which I used 0=Fool).
1s 2s 3s 4s 5s 6s 7s 8s 1c 2c 3c 4c 5c 6c 7c 8c
9p
1w 2w 3w 4w 5w 6w 7w 8w 1p 2p 3p 4p 5p 6p 7p 8p
They do NOT form more hexagons,
as they represent spinor representations
as opposed to vector representations,
so the final tarot pattern for E6 is
the D5 4-level nest of hexagons plus the 33:
Qp ks kw kc kp
16 17 18 19 js
7 8 9 20 jw
2 3 10 21 jc
1 6 15 Qc 0
4 5 14 Qw 9c
11 12 13 Qs 9w
Ks Kw Kc Kp 9s
jp 10s 10w 10c 10p
1s 2s 3s 4s 5s 6s 7s 8s 1c 2c 3c 4c 5c 6c 7c 8c
9p [ For another version click HERE. ]
1w 2w 3w 4w 5w 6w 7w 8w 1p 2p 3p 4p 5p 6p 7p 8p
E6 has dimension 78 = 45+32+1.
The 32 form a 16-dim complex space
with 16-dim real Shilov boundary.
Compare the 16 original members of the 18 Lohan.
Also, compare the two U(1)'s in the fibrations
E6 / (Spin(10)xU(1)) and Spin(10) / (Spin(8)xU(1))
with the two Lohan additional to the original 16
and
with two of the 9 Moving Stars additional to the 7 of the Big Dipper.
E6 is related to the Tai Hsaun Ching.
A 78-card Tarot Spread based on the hexagonal D4-D5-E6 structure is
O
O O
O O
O O O
O O O O
O O O O
O O O O O
O O O O O O
O O O O O O
O O O O O O O
O O O O O O O O
O O O O O O
O O O O O
O O O O
O O O O
O O O O
O O O O
O O
O
An alternative, but equally valid, way to build a
representation is to represent D5, not by nested hexagons,
but by the following triangle:
1 Spin(2)=U(1)
2 3 Spin(3)=SU(2)=Sp(1)=S3
4 5 6 Spin(4)=Spin(3)xSpin(3)
7 8 9 10 Spin(5) = Sp(2)
11 12 13 14 15 Spin(6) = SU(4)
16 17 18 19 20 21 Spin(7)
Ks Kw Kc Kp Qs Qw Qc Spin(8) = D4
Qp ks kw kc kp js jw jc Spin(9)
jp 10s 10w 10c 0 10p 9s 9w 9c Spin(10) = D5
A 78-card Tarot Spread based on the triangular D4-D5-E6 structure is
O
O O O O O O O O
O O O O O O O O
O O O O O O O
O O O O O O
O O O O O
O O O O O O
O O O O O
O O O O
O O O O O
O O O O
O O O O
O O O O
O O O O
O O
O O
O O
O
The entire spread represents 78-dimensional E6,
which can be constructed from
28+24 = 52-dimensional F4,
and
4+1+4+8+1+8 = 26-dimensional J3(O).
The minor arcana has 4x8=8x4=32 plus 3x8=6x4=24 cards.
The 78-card Triangular Spread is related to the
Tarot structure described around 1910 by P. D.Oupensky:
______________ | | | /\ | | / \ | | / * \ | | /______\ | | | |______________|
In Oupensky's structure:
Manly P. Hall considered the first 6 rows of the triangle
1 Spin(2)=U(1)
2 3 Spin(3)=SU(2)=Sp(1)=S3
4 5 6 Spin(4)=Spin(3)xSpin(3)
7 8 9 10 Spin(5) = Sp(2)
11 12 13 14 15 Spin(6) = SU(4)
16 17 18 19 20 21 Spin(7)
to represent the major arcana (not including 0=Fool) of the Tarot,
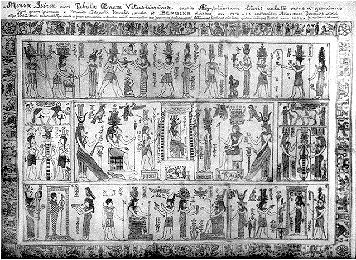
basing his conclusion upon study of the Bembine Tablet of Isis:
To see a more detailed 180k gif, click on the image. According to Hall, the bronze and silver Tablet was bought by Cardinal Bembo, who was historiographer of the Republic of Venice and later librarian of St. Marks, after the Sack of Rome in 1527. Following the death of Cardinal Bembo in 1547, the Tablet of Isis was kept in the museum of the House of Mantua until 1630, was exhibited in the Bibliotheque Nationale in Paris in 1809, and was last known to have been exhibited in the center of Gallery 2 of the Museum of Antiquities in Turin. The border of the Tablet
contains 4 corner figures plus 74 other figures, for a total of 78, the dimension of E6. Let the 4 corner figures represent the 4 Cartan subalgebra elements of E6 that are in its Spin(8) subalgebra. The rest of the top bar of the border contains 24 figures, which represent the vertices of a 4-dimensional 24-cell, the root vector polytope of 28-dimensional Spin(8). Compare the 28 Hsiu. The top full panel of the Tablet
contains 12 large figures and 2 small figures, and represents the 14-dimensional rank 2 Lie algebra G2, the Lie algebra of the automorphism group of the octonions. The two side central full panels of the Tablet
each contain a top level with 3 large and 1 small figures, and a bottom level with 3 large figures, and each represents a 7-dimensional sphere S7. Compare the 7 stars of the Big Dipper. Together, the top and side central full panels represent the fibre-product G2 x S7 x S7 = Spin(8) = D4:
The center central full panel contains 7 large figures,
and represents the 7-sphere that
represents the 7 imaginary octonions {i,j,k,E,I,J,K}:
The center part of the bottom full panel contains 8 large figures,
and represents the 8-dimensional octonions {1,i,j,k,E,I,J,K}:
Taken together, the center central and center bottom full panels represent the 7+8=15-dimensional 15-sphere,
which has a Hopf fibration into the 7-sphere and the 8-sphere. The two side bottom full panels
each contain one large figure separated from
the central part by a barrier, or large figure in a box.
The 2 large figures represent the {F,G} elements
of the 10-dimensional Sefirot algebra,
and the prominent separating figure-in-box figures indicate
that an important property (division algebra) is lost
when you go from the 8-dim octonions to
the 10-dim Sefirot algebra, which is then represented by
the entire bottom full panel:
----------------------------------------------------------- Another Egyptian representation of the Tarot may be found in the design of the Temple at Luxor, which also is related to the D4-D5-E6 physics model, and to sedenions.
References: The Tarot, by Cynthia Giles, Fireside (1992, 1994); The Encyclopedia of Tarot, vol. I, by Stuart R. Kaplan, U. S. Games Systems, Inc. (1978); The Isiac Tablet, by W. Wynn Westcott, The Philosophical Research Society, Inc. (1976); The Secret Teachings of All Ages, by Manly P. Hall, The Philosophical Research Society, Inc. (1988).
...
...