
Conway and Sloane (in their book Sphere Packings, Lattices, and Groups, Third Edition, Springer 199) say on page 119:
"... Formally we define Dn+ = Dn u ( [1] + Dn ). ... Dn+ is a lattice packing if and only if n is even. D3+ is the tetrahedral or diamond packing ... and D4+ = Z4. When n = 8 this construction is especially important, the lattice D8+ being known as E8 ...".] ...
According to John C. Baez and S. Jay Olson in their paper at gr-qc/0201030:
"... Ng and van Dam have argued that quantum theory and general relativity give a lower bound delta L > L^(1/3) L_P ^(2/3) on the uncertainty of any distance, where L is the distance to be measured and L_P is the Planck length. Their idea is roughly that to minimize the position uncertainty of a freely falling measuring device one must increase its mass, but if its mass becomes too large it will collapse to form a black hole. ... Amelino-Camelia has gone even further, arguing that delta L > L^(1/2) L_P ^(1/2) ... Here we show that one can go below the Ng-van Dam bound [ and the Amelino-Camelia bound ] by attaching the measuring device to a massive elastic rod. ...[ while the Ng-van Dam ] result was obtained by multiplying two independent lower bounds on delta L, one from quantum mechanics and the other from general relativity, ours arises from an interplay between competing effects. On the one hand, we wish to make the rod as heavy as possible to minimize the quantum-mechanical spreading of its center of mass. To prevent it from becoming a black hole, we must also make it very long. On the other hand, as it becomes longer, the zero-point fluctuations of its ends increase, due to the relativistic limitations on its rigidity. We achieve the best result by making the rod just a bit longer than its own Schwarzschild radius.
... Relativistic limitations on the rod's rigidity, together with the constraint that its length exceeds its Schwarzschild radius, imply that zero-point fluctuations of the rod give an uncertainty delta L > L_P . ...".
The Discrete HyperDiamond Generalized Feynman Checkerboard and Continuous Manifolds are related by Quantum Superposition:
Volumes of Spaces of Superpositions of other given Sets of Basis Elements correspond to Volume of Physical SpaceTime and Volume of Internal Symmetry Space represented by those Basis Elements.
The D4-D5-E5-E7-E8 VoDou Physics HyperDiamond Feynman Checkerboard model can be constructed from the 8-fold Periodicity of Real Clifford Algebras
where x ..N.. x denotes N-fold tensor product, by using a very large Clifford Algebra Cl(8N) as a starting point, and using 8-fold Periodicity to factor Cl(8N) into N copies of Cl(8).
To see what to do with the N copies of Cl(8), look at how Feynman's 2-dimensional Checkerboard might be constructed from N copies of Cl(2).
Given N copies of Cl(2), how can they be put in a useful order?Look at Cl(2) = C(2), with graded structure
1 2 1 The Cl(2) vector space is 2-dimensional, so the N copies of Cl(2) should be put in a 2-dimensional array. The most natural such array would be the Complex Gaussian integers, which make a 2-dim Feynman checkerboard with all the Cl(2) at the vertices of the checkerboard connected to other Cl(2) in such a way as to make a 2-dim lattice that is consistent with complex number multiplication.
Cl(2) has 1-dim U(1) as its bivector Lie algebra, which is consistent with the electromagntism gauge group, and with complex number multiplication, and Cl(2) has full spinor space of dimension sqrt(4) = 2, and so half-spinor spaces that are 1-dimensional, one for the electron and one for the positron to move around on the checkerboard, so a Complex Gaussian lattice with a Cl(2) at each vertex defines a 2-dimensional Feynman Checkerboard with half-spinor particles moving around on it and a U(1) gauge group providing the i that Feynman used to weight changes of direction.
If you look at the N copies of Cl(8) the same way, you see that Cl(8) has graded structure
so that Cl(8) has an 8-dim vector space, so that the N copies of Cl(8) wants to be connected as an 8-dim checkerboard, so the N copies of Cl(8) should be ordered as an array of integral octonions, that is, they should live on an E8 lattice, which is the Octonionic 8-dim correspondent of the 2-dim Complex Gaussian lattice.
You can look at the natural 4-dim physical spacetime sublattice, and see that it is the 4-dim HyperDiamond lattice.
Since Cl(8) half-spinors are 8-dim, you get (where Cl(2) gives you the electron and positron) for fermions to move on the lattice the 8 first generation fermion particles
and their 8 antiparticles. The projection of an 8-dim E8 lattice onto the 4-dim HyperDiamond lattice gives the second and third generation fermions.
At each vertex, instead of the Cl(2) gauge group U(1), you get the Cl(8) gauge group Spin(8), which gives Gravity and the Standard Model from the point of view of the 4-dim HyperDiamond lattice, and which is consistent with octonion multiplication.
The HyperDiamond generalization has discrete lightcone directions. If the 4-dimensional Feynman Checkerboard is coordinatized by the quaternions Q:
In cylindrical coordinates t,r with r^2 = x^2+y^2+z^2, the Euclidian metric is
and the Wick-Rotated Minkowski metric with speed of light c is
For the future lightcone links on the 4-dimensional Minkowski lightcone, c = sqrt3.
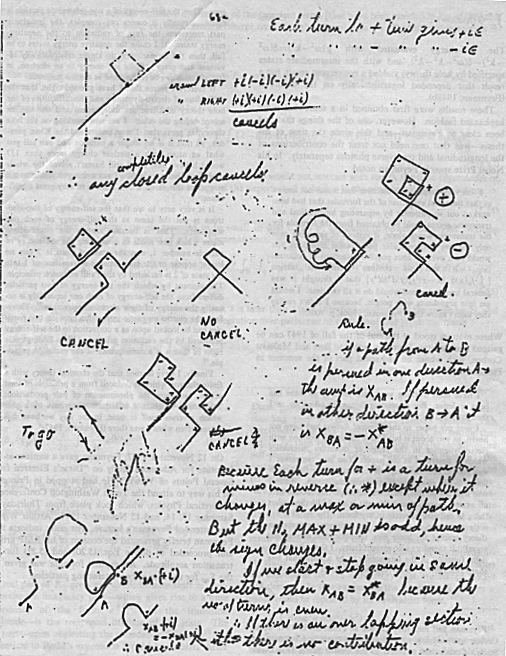
Any future lightcone link is taken into any other future lightcone link by quaternion multiplication by +/- i, +/- j, or +/- k.
For a given vertex on a given path, continuation in the same direction can be represented by the link 1, and changing direction can be represented by the imaginary quaternion +/- i, +/- j, +/- k corresponding to the link transformation that makes the change of direction.
Therefore, at a vertex where a path changes direction, a path can be weighted by quaternion imaginaries just as it is weighted by the complex imaginary i in the 2-dimensional case.
If the path does change direction at a vertex, then the path at the point of change gets a weight of -im e, -jm e, or -km e where i,j,k is the quaternion imaginary representing the change of direction, m is the mass (only massive particles can change directions), and sqrt3 e is the timelike length of a path segment, where the 4-dimensional speed of light is taken to be sqrt3.
For a given path, let C be the total number of direction changes, c be the cth change of direction, and ec be the quaternion imaginary i,j,k representing the cth change of direction.
C can be no greater than the timelike Checkerboard distance D between the initial and final points.
The total weight for the given path is then m sqrt3 ec to the Cth power times the product (c from 0 to C) of -ec
Note that since the quaternions are not commutative, the product must be taken in the correct order.
The product is a vector in the direction +/- 1, +/- i, +/- j, or +/- k.
Let N(C) be the number of paths with C changes in direction. The propagator amplitude for the particle to go from the initial vertex to the final vertex is the sum over all paths of the weights, that is the path integral sum over all weighted paths:
times
the Cth power of m sqrt3 ec
times
the product (c from 0 to C) of -ec
The propagator phase is the angle between the amplitude vector in quaternionic 4-space and the quaternionic real axis. The plane in quaternionic 4-space defined by the amplitude vector and the quaternionic real axis can be regarded as the complex plane of the propagator phase.
Conway and Sloane, in their book Sphere Packings, Lattices, and Groups (3rd edition, Springer, 1999).in chapter 4, section 7.3, pages 119-120) define a packing
[ where the gule vector [1] = (1/2, ... , 1/2) ] and say: "... D+n is a lattice packing if and only if n is even.
D+n is what David Finkelstein and I named a HyperDiamond lattice (although in odd dimensions it is technically only a packing and not a lattice).
Conway and Sloane also say in chapter 4, section 7.1, page 117) that the lattice Dn is defined only for n greater than or equal to 3.
To see what happens for n = 2, note that D2 should correspond to the Lie algebra Spin(4),which is reducible to Spin(3)xSpin(3) = SU(2)xSU(2) = Sp(1)xSp(1) = S3xS3, and is not an irreducible Lie algebra. The root lattice of D2 is two copies of the root lattice of SU(2), which is just a lattice of points uniformly distributed on a line.
If you are to fit the two lines together, you have to specify the angle at which they intersect each other, and requiring "lattice structure" or consistency with complex number multiplication does NOT unambiguously determine that angle: it can be either
60 degrees, which gives you the A2 root lattice
*--* / \ * * \ / *--*
of the Lie algebra SU(3) and the Eisenstein complex integers
90 degrees, which gives you the C2 = B2 root lattice
*--*--* | | * * | | *--*--*
of the Lie algebra Spin(5) = Sp(2) and the Gaussian complex integers.
Since a Dn lattice for n > 3 is a checkerboard, or half of a hypercubic lattice, it is natural to define D2 as a checkerboard, or half of a C2 = B2 square lattice. Then the 2-dimensional HyperDiamond lattice D+2 = D2 u ( [1] + D2 ) is seen to be the Z2 square lattice C2 = B2

where the orginal D2 is made up of the centers of the yellow squares, the glue vectors are the (1/2,1/2) represented by pairs of arrows, and the ( [1] + D2 ) is made up of the centers of the white squares.
The total 2-dim hyperdiamond structure is the Z2 integer lattice, sort of analogous to the 4-dim case in which D4 u ( [1] + D4 ) = Z4, so that
Note that the basic D2 structure is consistent with Feynman's 2-dimensional checkerboard in which the lines of the checkerboard are 2-dim light-cone lines.
Since the 4-dim HyperDiamond lattice is a 4-dim hypercubic lattice made up of two D4 lattices, one shifted by a glue vector ( 0.5, 0.5, 0.5, 0.5 ) with respect to the other, one of the D4 lattices can be regarded as the even sites of the full 4-dim HyperDiamond lattice.
Since the 4-dim HyperDiamond lattice is a 4-dim hypercubic lattice made up of two D4 lattices, one shifted by a glue vector ( 0.5, 0.5, 0.5, 0.5 ) with respect to the other, one of the D4 lattices can be regarded as the even sites of the full 4-dim HyperDiamond lattice.
If fermions live only on the even D4 sublattice, then hep-lat/9508013 by Kevin Cahill in the xxx e-print archive shows that the fermion doubling problem is solved.
The 4-dim HyperDiamond Feynman Checkerboard is closely related to Spin Networks. (Gersch (Int. J. Theor. Phys. 20 (1981) 491) has shown that the 2-dim Feynman Checkerboard is equivalent to the Ising model.)
In quant-ph/9503015 "Square Diagrams" are used to represent the 4-link future lightcone leading from a vertex because the representation is written in LaTeX code to be printed in black-and-white on 2-dimensional paper. The "true" representation of the 4-link future lightcone would be as a 4-dimensional simplex, with the 4 future ends of the links forming a 3-dimensional tetrahedron. It is shown here, with a stereo pair showing 3 dimensions and color coding (green = present, blue = future) for the 4th dimension.
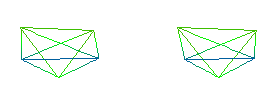
From the "true" representation, it is clear that the 4-link future lightcone leading from a vertex looks a lot like the Quantum Pentacle of David Finkelstein and Ernesto Rodriguez (Int. J. Theoret. Phys. 23 (1984) 887).
The future tetrahedron, not including the origin vertex, contains 4 vertices and 4 bivector triangles. Those bivectors correspond to the 4 translation bivectors of the 10-dimensional Spin(5) Lie algebra, which is based on the Cl(0,5) Clifford algebra with graded structure
1 5 10 10 5 1
so that there are: 1 empty set, 5 vector vertices, 10 bivector edges, 10 triangles, 5 tetrahedra, and 1 4-simplex. By Hodge duality, the 10 bivector edges correspond to the 10 triangles.
The future lightcone edges leading from the origin are edges on the 6 bivector triangles that include the origin vertex. Those bivectors correspond to the 6 bivectors of the Spin(4) subalgebra of the Spin(5) Lie algebra. The 6-dimensional Spin(4) subalgebra is reducible, isomorphic to SU(2) x SU(2), so that it reduces to 3 rotations and 3 Lorentz boosts.
The symmetries of the 4 future lightcone vectors can be seen from different points of view:
My viewpoint is to look at the 3-dimensional tetrahedron formed by the ends of the 4 future lightcone vectors. Its symmetry group is the 12-element tetrahedral group (3,3,2). The double cover of (3,3,2) is the 24-element binary tetrahedral group {3,3,2}. {3,3,2} is the group of unit quaternions in the 4-dim quaternionic lattice. The 24 unit quaternions in the 4-dim quaternionic lattice are the root vectors of the D4 Lie algebra Spin(8), from which I construct my D4-D5-E6-E7-E8 VoDou Physics model.
Another viewpoint is to look at the permutation group S4 of the 4 future lightcone vectors, then decompose S4 into subgroups, and then relate the subgroups of S4 to the groups of gravity and the standard model using a method of coherent states. This is the point of view of Michael Gibbs and David Finkelstein.
Still another viewpoint is to look at the permutation group S4 , then notice that S4 is the 24-element octahedral group (4,3,2), then use the McKay correspondence to get the Lie algebra E7, and then use E7 to build a physics model. This is the point of view of Saul Paul Sirag at the PCRG.
Yet another viewpoint, motivated by Spin Networks such as those described of Barnett and Crane, is to look at the dual to the 4-simplex illustrated above, in which its 5 vertices correspond to 5 tetrahedral 3-faces, and vice versa:
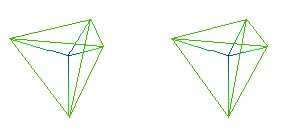
In this dual picture, the 4 vector edges leading from the origin break down into 3 green spacelike vector edges leading from the origin and 1 green-to-blue timelike vector edge leading from the origin. The 3 triangles with 2 spacelike sides correspond to the 3 rotations, and the 3 triangles with 1 spacelike side and 1 timelike side correspond to the 3 Lorentz boosts. As in the original lightcone picture, the 4 triangles that do not include the origin correspond to the 4 translations of the Spin(5) Lie algebra.
I hope, think, and believe that all these viewpoints are in a deep sense equivalent, and that we are really like several blind men trying to describe an elephant.
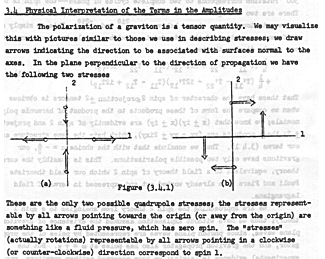
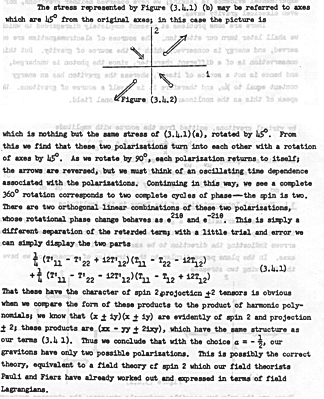
with his characteristic clarity in his "Lectures on Gravitation" (Caltech 1971). The following 2 gifs (63k and 90k) show relevant parts of pages 41 and 42:
In quant-ph/9503015 I considered only paths in which in each segment lies in the future lightcone, that is, in which time increased at each segment. Also, I used the Gersch convention of weighting changes in direction by -ime (where i is a quaternion imaginary).
Feynman also considered paths in which in each segment lies in the past lightcone, that is, segments going backward in time. He weighted the past lightcone changes in direction by the negative of the forward lightcone weight, which would be, using the Gersch convention, a weight by +ime (where i is a quaternion imaginary).
Feynman considered the path segments going backward in time to be antiparticle path segments. The following gif (300k) (from Schweber, Rev. Mod. Phys. 58 (1986) 449 at p. 482, (see box 13, folder 3, of Caltech's Feynman archives (Notes on the Dirac Equation))) shows Feynman's thinking:
see Conway and Sloane (Sphere Packings, Lattices, and Groups - Springer)
The complex Gaussian Z2 lattice, for which N(1)=N(2)=4, N(3)=0, N(4)=4, N(5)=8, ... and N(m)/4 is the number of distinguishable (i.e., 2^2 +2^2 = 8 indistinguishable, so N(8) = 4, and 2^2 + 1^2 = 5 = 1^2 + 2^2 distinguishable, so N(5) = 8) ways m can be written as the sum of 2 squares;
or
the complex Eisenstein lattice.
The 8-dimensional HyperDiamond octonionic E8 lattice is associated with the octonionic X-product of Cederwall and Preitschopf and a later paper of Dixon. For an E8 lattice N(m) is always less than N(m+1). Each vertex of an E8 lattice has 240 nearest neighbors and 2160 second-level next-nearest neighbors.
There are 7 distinct E8 lattices, denoted by iE8 (i = 1, ..., 7). All 7 of the E8 lattices have some points in common, and some subsets of three have some points in common, but no two of the E8 lattices are identical.
There are 7 distinct associative triangle 3-element subsets of
the 7 imaginary Octonions {i,j,k,E,I,J,K}.
There are 7 charged Dirac first-generation fermion particles:
At high energies, prior to Dimensional Reduction of SpaceTime, there is only one generation of fermions, so the first generation is the only generation. Therefore, each charged Dirac fermion particle, and its antiparticle, correspond to one imaginary Octonion, to one associative triangle, and to one E8 lattice:
red Down Quark red Up Quark green Down Quark Electron green Up Quark blue Down Quark blue Up Quark rD gD bD E rU gU bU I J K E i j k j / \ i---k J j J I J K / \ / \ / \ / \ / \ / \ i---K I---K I---k E---i E---j E---k 3E8 6E8 4E8 7E8 1E8 2E8 5E8
Since all the E8 lattices have in common the vertices { ±1, ±i, ±j, ±k, ±e, ±ie, ±je, ±ke }, all the charged Dirac fermions can interact with each other. Composite particles, such as Quark-AntiQuark mesons and 3-Quark hadrons, propagate on the common parts of the E8 lattices involved.
The uncharged e-neutrino fermion, which corresponds to the Octonion real axis with basis {1}, propagates on any and all of the E8 lattices.
After Dimensional Reduction of SpaceTime, the associative triangle of each E8 lattice is mapped into the same {i,j,k} Quaternionic associative triangle of the spatial part of 4-dimensional Physical SpaceTime, and the co-associative square of each E8 lattice is mapped into the {E,I,J,K} structure of 4-dimensional Internal Symmetry Space. There, the Generalized Feynman Checkerboard game is played on a 4-dimensional HyperDiamond Lattice.
The 16-dimensional Barnes-Wall lattice /\16, for which each vertex has 4,320 nearest neighbors.
4,320 = 480 + 3,840 = 2x240 + 16x240
The /\16 lattice is associated with the octonionic XY-product of Dixon.
TO REPRESENT THE 24-DIMENSIONAL SPACE THAT IS THE SUM OF THE 16-DIMENSIONAL FIRST GENERATION FERMION FULL-SPINOR SPACE AND THE 8-DIMENSIONAL VECTOR SPACETIME PRIOR TO DIMENSIONAL REDUCTION:
As described in a paper by Geoffrey Dixon, each vertex of the 24-dimensional Leech lattice /\24 has 196,560 nearest neighbors (norm(xx) = 4).
196,560 = 3x240 + 3x(16x240) + 3x(16x16x240)
Geoffrey Dixon is working on a book about the Leech lattice /\24.
The Conway group .0 (dotto) is the permutation group of the 196,560 vertices.
(See p. 295 of Conway and Sloane for connections among dotto, Fi24, M24, the binary Golay code C24, M12, and Suz.)
Note that the largest finite sporadic group, the Monster group, is the automorphism group of an algebra of dimension 196,884 = 196,560 + 300 + 24. (300 = symmetric tensor square of 24)
Also,
the 24-dimensional Leech lattice /\24 can be used to represent the 24 non-Abelian of the 28 Spin(8) gauge bosons.
The first person to propose Planck Scale Lorentz Invariance Violation as a solution to the problem of Ultra High Energy Cosmic Rays may have been L. Gonzalez-Mestres around 1995, prior to a proposal by Coleman and Glashow. Gonzalez-Mestres, in physics/0003080, gives his version of the history of the idea of Lorentz Invariance: "... Henri Poincare was the first author to consistently formulate the relativity principle, stating (Poincare, 1895): "Absolute motion of matter, or, to be more precise, the relative motion of weighable matter and ether, cannot be disclosed. All that can be done is to reveal the motion of weighable matter with respect to weighable matter". ... In his June 1905 paper (Poincare, 1905), published before Einsteins's article (Einstein, 1905) arrived (on June 30) to the editor, Henri Poincare explicitly wrote the relativistic transformation law for the charge density and velocity of motion and applied to gravity the "Lorentz group", assumed to hold for "forces of whatever origin". ... In 1921 , A. Einstein wrote in "Geometry and Experiment" (Einstein, 1921): "The interpretation of geometry advocated here cannot be directly applied to submolecular spaces... it might turn out that such an extrapolation is just as incorrect as an extension of the concept of temperature to particles of a solid of molecular dimensions". ...". Gonzalez-Mestres prefers a Quadratically Deformed Relativistic Kinematics ( QDRK )to the Linearly Deformed Relativistic Kinematics ( LDRK ) preferred by Amelino-Camelia and Piran in whose paper QDRK corresponds to the parameter value a = 2 and LDRK to a = 1. Gonzalez-Mestres says: "... QDRK naturally emerges when a fundamental length scale is introduced to deform the Klein-Gordon equa-tions. It is typical, for instance, of phonons in condensed-matter physics. ... LDRK can be generated by introducing a background gravitational field in the propagation equations of free particles. In the first case, the Planck scale is an internal parameter of the basic wave equations generating the "elementary" particles as vacuum excitations. In the second case, it manifests itself only as a parameter of the background gravitational field, similar to a refraction phenomenon. ...".
A December 1998 math.albany.edu eprint post from Laurent Siebenmann says:
"... censorship ... may seem to you, gentle reader, quite out of place in modern science. However, consider the case evoked below, where the preprint archive mp-arc is cited as offering evidence of unjust practice ...... Cordially
Laurent Siebenmann %%%%%%%%%%%%%%%%%%%%%%%%% ...
- From: Luis Gonzalez <Luis.Gonzalez@lapp.in2p3.fr>
- Message-Id: <199811241429.PAA26531@lapphp.in2p3.fr>
- Subject: The politics of Lorentz symmetry violation?
- To: math@math.polytechnique.fr
- Date: Tue, 24 Nov 1998 15:29:54 MET
Dear Colleague,
You have perhaps noticed that a paper presented at TAUP 97, "Lorentz symmetry violation and high-energy cosmic rays" (author: Luis Gonzalez-Mestres), did not appear in the conference Proceedings recently distributed by Elsevier. The paper exists, indeed, and has electronic dates at Los Alamos, APS, mp_arc... (first week of December 1997). It was also registered at KEK on December 12, 1997 and was, of course, sent to the editors in due time.
The local organizers actually refused to publish it, five months after it had been accepted and allocated three pages in the Proceedings. As you know, all papers selected for oral presentation were to be published in the Proceedings according to the official announcements, so why was my paper rejected by a late decision?
Looking at the Proceedings, I have found a paper by Sheldon L. GLASHOW on Lorentz symmetry violation, presenting two of my results without any mention to my work. These results are the possible absence of GZK cutoff and the stability of unstable particles at very high energy. Actually, such results had already been published electronically, but also from and editorial point of view, before the TAUP 97 conference was held. An example is my paper physics/9705031 (26 May 1997) of the Los Alamos archive, which was published in the Proceedings of ICRC 97 distributed at the end of July 1997, before the ICRC 97 conference started.
In the relevant region, the mechanisms producing the above mentioned effects are essentially identical in both approaches (that of Harvard and mine). However, I prefer the one I put forward because it automatically preserves Lorentz symmetry in the limit where k (wave vector) vanishes, and is likely to make things much easier in order to incorporate gravitation in the model. ...
... With my best regards
Luis Gonzalez-Mestres ...".
Here are some further
based on the properties of the D4 lattice, two copies of which make the 4HD HyperDiamond lattice. The D4 lattice nearest neighbor vertex figure, the 24-cell, is the 4HD HyperDiamond lattice next-to-nearest neighbor vertex figure.
Fermions move from vertex to vertex along links.
Gauge bosons are on links between two vertices, and so can also be considered as moving from vertex to vertex along links.
The only way a translation or rotation can be physically defined is by a series of movements of a particle along links.
A TRANSLATION is defined as a series of movements of a particle along links, each of which is the CONTINUATION of the immediately preceding link IN THE SAME DIRECTION.
An APPROXIMATE rotation, within an APPROXIMATION LEVEL D, is defined with respect to a given origin as a series of movements of a particle along links among vertices ALL of which are in the SET OF LAYERS LYING WITHIN D of norm (distance^2) R from the origin, that is, the SET OF LAYERS LYING BETWEEN norm R-D and norm R+D from the origin.
Conway and Sloane (Sphere Packings, Lattices, and Groups - Springer) pp. 118-119 and 108, is the reference that I have most used for studying lattices in detail.
(Conway and Sloane define the norm of a vector x to be its squared length xx.)
In the D4 lattice of integral quaternions,
layer 2 has the same number of vertices as layer 1, N(1) = N(2) = 24.
Also (this only holds for real, complex, quaternionic, or octonionic lattices), K(m) = N(m)/24 is multiplicative, meaning that, if p and q are relatively prime, K(pq) = K(p)K(q).
The multiplicative property implies that:
K(2^a) = K(2) = 1 (for a greater than 0) and
K(p^a) = 1 + p + p^2 + ... + p^a (for a greater than or equal to 0).
So, for the D4 lattice,
there is always an arbitrarily large layer (norm xx = 2^a, for some large a) with exactly 24 vertices, and
there is always an arbitrarily large layer(norm xx = P, for some large prime P) with 24(P+1) vertices (note that Mersenne primes are adjacent to powers of 2), and
given a prime number P whose layer is within D of the origin, which layer has N vertices, there is a layer kP with at least N vertices within D of any other given layer in D4.
Some examples I have used are chosen so that the 2^a layer adjoins the prime 2^a +/- 1 layer.
If you consider the D4 lattice to be the even sublattice of the 4-dimensional HyperDiamond lattice of the D4-D5-E6-E7-E8 physics model, then the minimal norm of the D4 lattice would be 2, and you would have a table in which the entries of the first column (m=norm of layer) are each twice the entries below, so that, for example, the layer of norm 22 with respect to the origin of the HyperDiamond lattice would have 288 vertices. This is the first definition (equation 86) of the D4 lattice in Chapter 4 of Sphere Packings, Lattices, and Groups, 3rd edition, by Conway and Sloane (Springer 1999). Conway and Sloane denote the HyperDiamond structure of dimension n by "the packing Dn+", because Dn+ is a lattice only if and if the dimension n is even (since Conway and Sloane define Dn only for n at least 3) .
The D4+ 4-dimensional HyperDiamond lattice is exactly the same as the 4-dimensional cubic lattice Z4, the lattice in 4-dimensional space made up of 4-dim hypercubes.
Conway and Sloane (Chapter 4, eq. 49) give equations for the number of vertices N(m) in the m-th layer of the D4+ HyperDiamond lattice:for m odd: N(m) = 8 SUM(d|m) d for m even: N(m) = 24 SUM(d|m, d odd) d
where d is a divisor ( including 1 and m ) of m.
The coincidence between D4+ and Z4 is peculiar to 4-dimensions. For example, D3+ is the familiar 3-dim diamond lattice, and D8+ is the E8 lattice, and they are not cubic lattices.
Conway and Sloane (Chapter 4, eq. 49, and eq. 102) give equations for the number of vertices N(m) in the m-th layer of the D8+ HyperDiamond lattice and of the E8 lattice:for D8+: N(m) = 16 SUM(d|m) d^3 for E8 and m odd, N(m) = 0
for E8 and m even, N(m) = 240 SUM(d|(m/2)) d^3
where d is a divisor ( including 1 and m / 2 ) of m / 2. For E8, N(m) is the number of integral octonions of norm m / 2.
The HyperDiamond lattice D4+ is made up of two D4 lattices. One of the D4 lattices in the D4+ is called the even D4 of D4+. If you start from the origin of D4+, the even D4 contains the layers that are even distances from the origin.
Since the D4 lattice is the lattice of integral quaternions, the even D4 of D4+ is the integral quaternion lattice expanded by a factor of 2 so that each layer is twice as far from the origin (and, in particular, the closest layer is at distance 2 instead of 1 from the origin).
Here are the numbers of vertices in some of the layers of the D4+ lattice. The even-numbered layers correspond ot the even D4 sublattice:
m=norm of layer N(m)=no. vert. 0 1 1 8 = 1 x 8 2 24 = 1 x 24 3 32 = ( 1 + 3 ) x 8 4 24 = 1 x 24 5 48 = ( 1 + 5 ) x 8 6 96 = ( 1 + 3 ) x 24 7 64 = ( 1 + 7 ) x 8 8 24 = 1 x 24 9 104 = ( 1 + 3 + 9 ) x 8 10 144 = ( 1 + 5 ) x 24 11 96 = ( 1 + 11 ) x 8 12 96 = ( 1 + 3 ) x 24 13 112 = ( 1 + 13 ) x 8 14 192 = ( 1 + 7 ) x 24 15 192 = ( 1 + 3 + 5 + 15 ) x 8 16 24 = 1 x 24 17 144 = ( 1 + 17 ) x 8 18 312 = ( 1 + 3 + 9 ) x 24 19 160 = ( 1 + 19 ) x 8 20 144 = ( 1 + 5 ) x 24 21 256 = ( 1 + 3 + 7 + 21 ) x 8 22 288 = ( 1 + 11 ) x 24 23 192 = ( 1 + 23 ) x 8 24 96 = ( 1 + 3 ) x 24 25 248 = ( 1 + 5 + 25 ) x 8 26 336 = ( 1 + 13 ) x 24 27 320 = ( 1 + 3 + 9 + 27 ) x 8 28 192 = ( 1 + 7 ) x 24 29 240 = ( 1 + 29 ) x 8 30 576 = ( 1 + 3 + 5 + 15 ) x 24 31 256 = ( 1 + 31 ) x 8 32 24 = 1 x 24 33 384 = ( 1 + 3 + 11 + 33 ) x 8 34 432 = ( 1 + 17) x 24 35 384 = ( 1 + 5 + 7 + 35 ) x 8 36 312 = ( 1 + 3 + 9 ) x 24 37 304 = ( 1 + 37 ) x 8 38 480 = ( 1 + 19 ) x 24 39 448 = ( 1 + 3 + 13 + 39 ) x 8 40 144 = ( 1 + 5 ) x 24 41 336 = ( 1 + 41 ) x 8 42 768 = ( 1 + 3 + 7 + 21 ) x 24 43 352 = ( 1 + 43 ) x 8 44 288 = ( 1 + 11) x 24 45 624 = ( 1 + 3 + 5 + 9 + 15 + 45) x 8
Note that layer 22 with 288 vertices follows layer 21 with ( 1 + 3 + 7 + 21 ) x 8 = 256 = 16x16 = 2^8 vertices.
The notation in the following table is based on the minimal norm of the D4 lattice being 1, in which case the D4 lattice is the lattice of integral quaternions. This is the second definition (equation 90) of the D4 lattice in Chapter 4 of Sphere Packings, Lattices, and Groups, 3rd edition, by Conway and Sloane (Springer 1999), who note that the Dn lattice is the checkerboard lattice in n dimensions.
m=norm of layer N(m)=no. vert. K(m)=N(m)/24 1 24 1 2 24 1 3 96 4 4 24 1 5 144 6 6 96 4 7 192 8 8 24 1 9 312 13 10 144 6 11 288 12 12 96 4 13 336 14 14 192 8 15 576 24 16 24 1 17 432 18 18 312 13 19 480 20 20 144 6 127 3,072 128 128 24 1 65,536=2^16 24 1 65,537 1,572,912 65,538 2,147,483,647 51,539,607,552 2,147,483,648 2,147,483,648=2^31 24 1
65,537 = 2^2^4 + 1 is the largest known Fermat prime. It is called F4, but is not likely to be confused with the exceptional Lie algebra F4.
2,147,483,647 = 2^31 - 1 is a Mersenne prime. It was shown to be prime by Euler. It is called M31, but is not likely to be confused with the Andromeda galaxy M31.
(see Wells, The Penguin Dictionary of Curious and Interesting Numbers, Penguin, 1986)
If the D4 spacetime lattice length is taken to be the Planck length, about 10^-33 cm or, in terms of energy, about 10^19 GeV, then
the layer of norm 65,537 is at a distance sqrt(65,537) = 256.002 x 10^-33 cm or about 3.9 x 10^16 GeV, and
the layer of norm 2,147,483,647 is at a distance sqrt(2,147,483,647) = 46,340 x 10^-33 cm or about 2.2 x 10^14 GeV, and
THEREFORE
at energies below about 10^16 GeV, continuous rotations can be approximated by D4 lattice rotations to an accuracy of at least 2 PI^2 / 1,572,912 = about 1.3 x 10^-5 steradians(4-dim), and
at energies below about 10^14 GeV, continuous rotations can be approximated by D4 lattice rotations to an accuracy of at least 2 PI^2 / 51,539,607,552 = about 3.8 x 10^-10 steradians(4-dim).
The argument can be extended quite a long way by considering the Mersenne prime 2^859433-1 (258716 digits) found by Slowinski and Gage in 1994.
THEREFORE
IT IS UNLIKELY THAT PLANCK-LENGTH D4 LATTICE SPACETIME IS PRESENTLY EXPERIMENTALLY DISTINGUISHABLE FROM CONTINUOUS SPACETIME BY DIRECT OBSERVATION OF ROTATIONS.
Richard Feynman said, in his book QED, Princeton 1985 at page 129:
Feynman got a nice representation of Dirac physics in 2-dim by using a 2-dim lattice that is really the Gaussian integer lattice in the Complex plane. His generalization to 4-dim was:
Details of Feynman's unproductive line of thought are in Schweber's book, QED, Princeton 1994, pages 406-407: "... Feynman showed that one could derive the Dirac equation in one-space-one-time dimension if the amplitude for a path with R corners is taken to be (i m epsilon)^R. Stated differently, each time the electron reverses spatial direction, it acquires a phase factor e^(i pi / 2) ... Feynman encountered difficulties in extending the idea of loading each turn through e^(i theta / 2) which worked in one-space dimension to higher dimensions because in those situations the angles theta are in different planes. He tried to use quaternions and octonions (quaternions representing euclidean 4-dimensional rotations) to represent wave functions, but he was not able to obtain a natural representation of the Dirac equation as an integral over path. ...".
More details are in his letter to Welton of 10 February 1947 (back in 1986, a friend of mine got a copy from the Caltech archives), in which Feynman says: "... we have at the point ... in the path to keep track of a rotation in three space (around the axis of the tangent to the path) ... when I studied quaternions which I knew were designed to represent rotations I realized that they were the mathematical tool in which to represent my thoughts. ... My purpose now is to consider a path as a set of four functions Xm(s) = X,Y,Z,T(s) of a parameter s. Thus the speed need not be that of light ... The idea now is to consider a path is a succession of 4vectors Vm(1), Vm(2), Vm(3) .. representing successive proper velocities (V_m V^m = 1) on the path. Then each path represents a net Lorentz-Rotation transformation. (I mean a combination of a Rot. and a Lorentz.) ... We desire a symbolism, which by analogy works in 4(3+1)space like the quaternions do in 3. I shall show that the symbolism is furnished by quaternions using complex numbers for components! ... (lets call them octonions) ... Thus, a octonion is a quaternion whose coeficients are of the form a + Rb. In 3+1 space R^2 = -1 and we can take R = i if we wish, so octonions for Lorenzt Trans. and Rotations are quaternions with complex (a + bi) coefficients. QED. ..."
Feynman gives an octonion basis that looks like the conventional one: if the quaternion basis is {1,i,j,k}, then, if you let R = E, Ri = I, Rj = J, and Rk = K, you get for his basis {1,i,j,k,R,Ri,Rj,Rk} which looks like a conventional {1,i,j,k,E,I,J,K}.
However, Feynman does NOT use the octonions to represent an 8-real-dimensional vector space: rather,
Feynman uses octonions to represent Rotations (by the {i,j,k}) and Lorentz transformations (by the {Ri,Rj,Rk}).
That is related to a technical problem with Feynman's statement "... we can take R = i if we wish ... so octonions ... are quaternions with complex (a + bi) coefficients ..."
What I (and most other people) call octonions are NOT complexified quaternions, but are a division algebra over the REAL number field. Therefore, R is NOT to be identified with the Complex imaginary i.
In fact, the i in the basis {1,i,j,k,R,Ri,Rj,Rk} is the Complex imaginary i, because {1,i} is a basis for the Complex subalgebra of the quaternions and of the Octonions, so the role of the Complex imaginary i is already filled.
In conventional math, the complexified quaternions (biquaternions, tensor product CxQ) DO exist, but they are just the algebra of 2x2 Complex matrices, GL(2,C), so Feynman reinvented in his own way the result that the Lorentz transformations (including Rotations) are in GL(2,C), and since Feynman's discussion could be restricted to unit modulus,
Feynman's reinvention is really that the Lorentz transformations (including rotations) are SL(2,C), or, in conventional math language, that SO(3,C) is isomorphic to SL(2,C).
His mathematical result is correct (even though his terminology is NOT consistent with normal math terminology), but it did not help him at all with his physics problem, which was generalizing his 2-dim Feynman Checkerboard to higher dimensions.
He would have found that 4-dim Quaternions don't quite work for all 4 forces of physics, but that the 8-dimensional Octonionic lattice will give you all 4 forces of physics, and it naturally breaks down into a 4-dim HyperDiamond physical spacetime lattice and an internal symmetry lattice, which is the HyperDiamond lattice version of the D4-D5-E6-E7-E8 VoDou Physics model.
I did not fully realize what Feynman should have done until about a year after I saw (in 1986) a copy of his 10 Feb 1947 letter to Welton, and then I did not understand my own stuff as well as I do now. Then (in 1987) I went to Caltech to tell Feynman that he should look at discrete 4-dim quaternionic and 8-dim octonionic integers as the proper generalization of his Gaussian Complex Feynman Checkerboard lattice. He did not know me, and I just went to his office at Caltech. He was there, but Helen Tuck told me to go away, so I did. Later I realized that my visit was shortly before his 4th cancer operation and only about half a year before his death in early 1988, so that he was really quite sick and in a good deal of pain, so she was being very protective of him.
......