WHERE DO THE 480 OCTONION MULTIPLICATION PRODUCTS COME FROM?
One point of view is based on Clifford Algebras.
This point of view is based on the paper
of Jorg Schray and Corinne Manogue, hep-th/9407179
Octonionic Representations of Clifford Algebras and Triality
and the book of H. S. M. Coxeter,
Complex Regular Polytopes, 2nd ed, Cambridge 1991
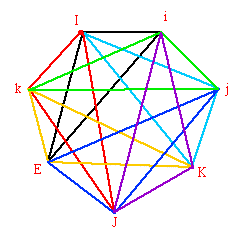
Start with the 7 imaginary octonions i, j, k, E, I, J, K.
This includes 1, since ii = jj = ... = -1.
You have 2^7 = 128 sign changes.
You have 7! = 2x3x4x5x6x7 permutation changes.
However, all 128 x 7! changes do NOT
give different multiplication tables.
Of the 128 sign changes,
the 2^3 = 8 changes of i, j, and E
do NOT give a different multiplication.
Of the 7! permutation changes, those preserving the group
PSL(2,7) = SL(3,2) do NOT give a different multiplication.
The order of PSL(2,7) is 2^3 x 3 x 7 = 168.
It is a simple group of Lie type denoted A1(7) = A2(2),
and can be thought of as the group of
linear fractional transformations of the vertices of
a heptagon (Chinese Horoscope figure)
(the vertices representing Z7).
It is related to a 7-coloring of triangles in hyperbolic plane,
meeting at 7 at a vertex and colored so that no two triangles
at a given vertex share a color,
and to a 7-coloring of a 3-holed torus formed by identifying
sides 2k+1 and 2k+6 (mod 14) in this figure:
(see Dana Mackenzie's article
in American Mathematical Monthly (Oct 95) 706-715.)
Therefore the number of different multiplications due
to sign changes and permutations is:
128 x 2 x 3 x 4 x 5 x 6 x 7 / 8 x 2 x 2 x 2 x 3 x 7 =
= 16 x 5 x 6 = 480
The 480 multiplications are made up of two sets of 240 each,
a product in one set being
found in the reverse order in the other set.
The two sets of 240 multiplications are called
sets of opposite multiplications.
Note that you can get an opposite multiplication
for quaternions:
from ij = k you can reverse to get ji = k,
which is an opposite multiplication.
Complex numbers, with only the one imaginary i,
have only one multiplication.
---------------------------------
What is PSL(2,7) ?
PSL(2,7) is the 168-element simple group that is
the central quotient group of SL(2,7).
SL(2,7) is the 336-element group of 2x2 matrices
with determinant 1
whose entries are elements of the finite group Z7 .
Z7 is just the integers mod 7, that is,
0, 1, 2, 3, 4, 5, 6 with the identification 7=0.
If Z7 is represented by the vertices of a heptagon,
then PSL(2,7) is the linear fractional group of the
vertices of the heptagon.
---------------------------------
PSL(2,7) corresponds to the Fano projective plane.
Burkland Polster, in his article in The Mathematical Intelligencer 21 (1999) 38-43, shows the Fano plane from a stereo 3-dimensional point of view
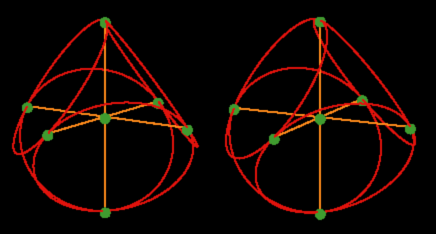
in which it is formed by the 6 vertices of an octahedron plus its center, with lines being the 3 diagonals of the octahedron plus the 4 circles which circumscribe 4 of the 8 faces of the octahedron. The resulting figure is what Arthur Young calls a heptaverton. It has the symmetry group of the octahedron,which is or order 24. Burkland Polster also shows the Fano plane from a very symmetrical point of view
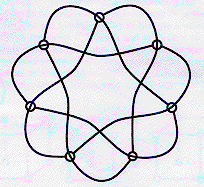
with cyclic symmetry group of order 7.
As Burkland Polster points out, the order of total symmetry group of the Fano plane PSL(2,7) is then seen to be the product of the orders of those two figures, 24 x 7 = 168.
The symmetry of the binary double-cover of the octahedral group, of order 48, would give a total order of 48 x 7 = 336, the order of Sl(2,7).
---------------------------------
Instead of the group PSL(2,7),
Schray and Manogue use the isomorphic group SL(3,2),
which they denote PGL(3,Z2).
They view it as the group of the Fano projective plane
over the field Z2 with two elements, and
they use the following diagram,
which is the more common representation of the Fano plane,
to represent octonion multiplication:

The Geometry of the Fano Plane configuration also gives the Golden Ratio, as described on this image

from a
Bruce A. Rawles web page. The Golden Ratio comes from sqrt(5) which
in turn comes from the fact that AC = FC is 4 times CD so that by
Pythagorean theorem AD is sqrt(15) times CD and
sqrt(15) factors into sqrt(3) times sqrt(5) which gives Golden Ratio.
If you also put a circle inscribed in the large triangle
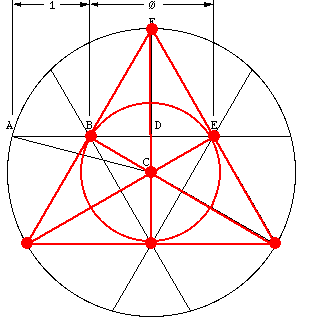
you have 7 points each at an intersection of 3 lines and 7 lines
each containing 3 points
which is the Fano configuration 7_3 which is the Fano projective plane
which has
the 168-element symmetry of PSL(2,7) = SL(3,2).
The 7 points of the Fano plane represent the 7 imaginary octions and
the 168 symmetries are permutation symmetries that preserve an octonion
multiplication table.
According to a web page at
home.wlu.edu/~mcraea/finite_geometry/projectivegeometry/prob28configurations/problem28.htm
:
"... start with ... the Fano plane (each line has its own color) ...

... and then ... place its 7 points equally spaced along a circle.
We then represent the lines as triangles ...
... and it is easy to see that the automorphism group of the Fano
plane contains the isometry group of the regular 7-gon (remember that
the automorphism group of the Fano plane contains 168 elements, while
that of
the dihedral group D7 contains 14 elements). ...[
If you replace the Fano red circle (1 line) with a triangle (3
lines)
and replace the Fano center point with 3 points (one on each of the new
triangle lines)
then you transform the unique Fano 7_3 configuration of 7 lines and
points each containing 3 of the other
to one of the three 9_3 configurations of 9 lines and points each
containing 3 of the other ]...
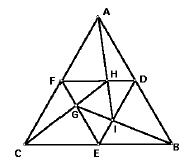
...[ in which the new points GHI replace FDE as the terminators of
lines starting at BCA
and, with FDE at midpoints of the edges of the triangle BCA, the ratio
HD / HF is the Golden Ratio.
The three triangles ABC, DEF, and GHI correspond to the Jittterbug
Transformation
of triangular faces of the Octahedron, Cuboctahedron, and Icosahedron.
If in the 9_3 ]... we connect two points eactly when they are not
connected by a line ...[ we get ]...
 ...[ which generalizes the 7_3 figure ]...
...[ which generalizes the 7_3 figure ]... 
As for the Fano 7_3 plane, Wikipedia states:
"... There are 7 points, and 24 symmetries fixing any point.
There are 7 lines, and 24 symmetries fixing any line.
There are 21 unordered pairs of points, each of which may be mapped by
a symmetry
onto any other unordered pair. For any unordered pair there are 8
symmetries fixing it.
There are 21 flags consisting of a line and a point on that line. Each
flag corresponds
to the unordered pair of the other two points on the same line.
For each flag, 8 different symmetries keep it fixed.
There are 21 ways of selecting a quadrangle of four cyclically ordered
points
no three of which are collinear, and eight symmetries that fix any such
quadrangle.
For each flag consisting of a point p and a line l, there is a unique
quadrangle in which
the four points of the quadrangle are disjoint from l and
the four edges of the quadrangle do not pass through p,
and every quadrangle corresponds to a flag in this way.
There are 28 triangles, which correspond one-for-one with the 28
bitangents of a quartic ...
For each triangle there are six symmetries fixing it,
one for each permutation of the points within the triangle.
There are 28 ways of selecting a point and a line that are not incident
to each other,
and six ways of permuting the Fano plane while keeping a configuration
of this type fixed.
For every non-incident point-line pair (p,l),
the three points that are unequal to p and that do not belong to l form
a triangle,
and for every triangle there is a unique way of grouping the
remaining four points into a non-incident point-line pair.
There are 28 ways of specifying a hexagon in which no three consecutive
vertices lie on a line,
and six symmetries fixing any such hexagon.
There are 42 ordered pairs of points,
and again each may be mapped by a symmetry onto any other ordered pair.
For any ordered pair there are 4 symmetries fixing it.
There are 84 ways of specifying a triangle together with one point on
that triangle,
each of which has two symmetries fixing it.
There are 84 ways of specifying a pentagon in which no three
consecutive vertices lie on a line,
and two symmetries fixing any pentagon.
There are 168 different ways of specifying a triangle together with an
ordering for its three points,
and only the identity symmetry fixes this configuration. ...".
E8 (248dim) = F4 (52dim) + 7 x D4 (28dim) = 8x31
E8 (240rootvectors) = F4 (48rootvectors) + 8 x D4 (24rootvectors) =
8x30
E8 (240rootvectors) = E6 (72rootvectors) + 7 x D4 (24rootvectors) =
E6 (72) + Fano (168)
Laurent Manivel in math/0507118 said:
"... the 27 lines on a complex cubic surface or the 28 bitangents
to a smooth quartic plane curve
have ... close connections with the Weyl groups of the root systems E6
and E7 ... through the
relation with Del Pezzo surfaces of degree three and two, respectively
...
Del Pezzo surfaces of degree one provide a similar identification of
the diameters of the root system E8,
with the 120 tritangent planes to a canonical space curve of genus 4.
...
The 27 lines on the cubic surface are in natural correspondence
with the weights of the minimal representation of E6 ...
Subalgebra Spin(10) ... correspondence [with]... Subconfiguration ...
Line ...
Subalgebra Spin(8) ... correspondence [with]... Subconfiguration
...Tritangent plane ...
...
the 28 bitangents to the quartic plane curve ... can be put in
correspondence
with pairs of opposite weights of the minimal representation of E7 ...
The 28 bitangents to a smooth plane quartic curve give rise
to 56 lines on the Del Pezzo surface of degree two
defined as the double cover of the projective plane, branched over the
quartic ...
There is an equivariant correspondence between the 28 triangles in the
Fano plane,
and the 28 pairs of points in the projective line over F7. ...
e7 and its minimal representation ... turn... out to be closely related
with the Fano plane
and the octonionic multiplication. ...
attach to each line L of the Fano plane a two dimensional vector space
AL.
Then we can describe e7 ... as
e7 (133dim) = 7 x { SU(2) (3dim) } + 7 x {(Aa x Ab x Ac x Ad) (abcd not
on L) (16dim)}
... e7 ... appear[s] as plane projective geometr[y] whose points are
copies of so8 ...
and whose lines are copies of ... so12 ...
...
For E8 the minimal representation is the adjoint one and is no longer
minuscule. ...
The bicanonical model of a Del Pezzo surface of degree one is the
double cover of a quadratic cone,
branched over a canonical space curve of genus 4 and degree 6 given by
the complete intersection of the cone with a unique cubic surface ...
The 240 lines on the Del Pezzo surface arise in pairs
from the 120 tritangent planes to the canonical curve ...
The automorphism group of the 240 lines is the Weyl group W(E8) of the
root system of type E8.
Its order is 696 729 600 = 128×27×5×8! = 2^13 3^5 5^2
7. ...
e8 ... appear[s] as plane projective geometr[y] whose points are copies
of so8 x so8 ...
and whose lines are copies of ... so16 ...
e8 = spin16 + D where D is a half-spin representation, of dimension
128. ...
when we extract from spin16 two orthogonal copies of spin8 ... we get
...[
e8 = spin8 x spin8 + (O1 x O1') + (O2 x O2') + (O3 x O3')
]... where O1, O2, O3 are the three eight-dimensional irreducible
representations of spin8.
This models splits the 120 tritangent planes into two groups of 12 and
three groups of 32. ...".
In summary, there are 8 x 168 = 1,344 symmetries that preserve an octonion product multiplication table. Since the total number of possible sign and permutation symmetries is 128 x 7! = 645,120, you can see that there are 645,120 / 1,344 = 480 octonion product multiplication tables. If you disregard the permutation symmetries, you have 128 / 8 = 16 products. If you disregard the sign symmetries, you have 7! / 168 = 5,040 / 168 = 30 products. Of the 30 octonions products (disregarding sign), 2 of the product rules are cyclic and dual to each other: e(n) * e(n+1) = e(n+3) e(n) * e(n+1) = e(n+5) The 30 octonion products (disregarding sign) may be regarded as 15 pairs of products, with the products in each pair dual to each other. One of the pairs is cyclic. If the 15 pairs correspond to the 15 sedenion imaginaries,
with basis _ _ _ _ _ _ _ _
i j k E I J K e i j k E I J K
then _
the cyclic pair may correspond to the "mirroring" imaginary e.
The 28 octonion products (disregarding sign)
may give rise to the 28 new associative triples of the sedenions.
Onar Aam uses the cyclic product e(n) * e(n+1) = e(n+3)
to show how to visualize the PSL(2,7) = GL(3,2) symmetries
that preserve a given octonion product multiplication table.
His method gives rise to a natural construction of a 3-dimensional
Start with three things:
480 different multiplication tables for octonion PRODUCTs;
8 = 2^3 SIGN CHANGES (of i, j, and E) that do NOT affect the product;
and
168 = 7x3x8 = 21x8 PERMUTATIONS that do NOT affect the product.
The 168 permutations form the PSL(2,7) = SL(3,2) symmetry group
of the octonion product multiplication table,
represented by the heptagon diagram or the triangle diagram.
To see explicitly how the permutations work,
start with these 7 associative 3-rings:
e0 * e1 = e3
e1 * e2 = e4
e2 * e3 = e5
e3 * e4 = e6
e4 * e5 = e0
e5 * e6 = e1
e6 * e0 = e2
Since cyclic permutations of the 3 elements of the 3-rings
do NOT change the product multiplication table,
you have 7x3 = 21 permutations that do NOT change the product.
That accounts for the factors 3 and 7 of 168 = 7x3x8.
To see where the factor 8 comes from,
consider the coassociative squares corresponding to each of the 3-rings.
For each square, if you permute any 3 of its 4 elements cyclically
you do NOT change the multiplication table.
The factor 8 in 168 = 7x3x8 comes from
the 8 nontrivial permutations of 4 elements of the form (123).
Note that
the 8 nontrivial permutations of 4 elements of the form (123)
are contained in S4, the group of permutations of 4 elements,
which is the octahedral group of order 24 = 4! = 3x8,
which is a subgroup of PSL(2,7) of order 168 = 7x24 = 7x3x8.
Now, arrange the 7 octonions in a heptagonal ring:
e0->e1->e2->e3->e4->e5->e6->e0
Then, make two more rings:
e0->e2->e4->e6->e1->e3->e5->e0
0 2 4 6 8 10 12 14 (mod7)
and
e0->e4->e1->e5->e2->e6->e3->e0
0 4 8 12 16 20 24 28 (mod7)
These 3 rings correspond to multiplying each index by:
2^0 = 1 = 1 (mod7)
2^1 = 2 = 2 (mod7)
2^2 = 4 = 4 (mod7)
Since 2^3 = 8 = 1 (mod7), you can only construct 3 rings that way.
Denote the 3 rings by R(n), R(2n), and R(4n).
They all correspond to the SAME product.
For instance, look at the R(n) term e1 * e2 = e4
When you go to R(2n), it becomes e2 * e4 = e1
When you go to R(4n), it becomes e4 * e1 = e2
Or, look at the R(n) term e0 * e1 = e3
When you go to R(2n), it becomes e0 * e2 = e6
When you go to R(4n), it becomes e0 * e4 = e5
In each case,
the R(2n) and R(4n) products are in the SAME multiplication
table as the R(n) multiplication table.
That enables you to combine R(n), R(2n), and R(4n) to
make ONE onarhedral lattice
(Onar's diagram sort of reminds me of the scene in the movie Contact
where the Helluvan Engineer describes how to think of the code
in 3-dimensional terms, not 2-dimensional):
e3-e4-e5-e6-e0-e1-e2
/ / / / / / /
e6-e0-e1-e2-e3-e4-e5
/ / / / / / /
e2-e3-e4-e5-e6-e0-e1
/ / / / / / /
e5-e6-e0-e1-e2-e3-e4
/ / / / / / / z-axis index = 4n
e1-e2-e3-e4-e5-e6-e0 _.
/ / / / / / / /|
e4-e5-e6-e0-e1-e2-e3 /
/ / / / / / / /
e0-e1-e2-e3-e4-e5-e6 +-------------> x-axis index = n
| | | | | | | |
e2-e3-e4-e5-e6-e0-e1 |
| | | | | | | |
e4-e5-e6-e0-e1-e2-e3 \|/ y-axis index = 2n
| | | | | | |
e6-e0-e1-e2-e3-e4-e5
| | | | | | |
e1-e2-e3-e4-e5-e6-e0
| | | | | | |
e3-e4-e5-e6-e0-e1-e2
| | | | | | |
e5-e6-e0-e1-e2-e3-e4
An onarhedral lattice defines a tiling of 3-dimensional space.
Their PRODUCTS look like their LATTICES which
in turn look like the SYMMETRIES OF A PRODUCT.
All this is manifested in many ways, including
INFORMATION THEORY,
the STRUCTURE OF THE D4-D5-E6-E7-E8 VoDou Physics Model,
the OCTONION MIRRORHOUSE, and
OCTONION FRACTALS.
PRODUCTS = LATTICES = SYMMETRIES OF A PRODUCT:
1. MULTIPLICATION PRODUCTS:
We have just seen that for the octonions,
with 7 imaginaries i, j, k, E, I, J, K
there are 240 octonion products
plus another 240 opposite octonion products.
Octonion products correspond to E8 lattices.
Quaternion Products correspond to D4 lattices.
Complex Products correspond to Gaussian and Eisenstein lattices.
Real Products correspond to the lattice of Natural Numbers.
2. DISCRETE E8 LATTICES:
There are 7 E8 lattice integral octonion domains.
(1-1 correspondence with
the 7 imaginary octonions i,j,k,E,I,J,K)
Each E8 lattice has 2x24 + 8x24 = 48 + 192 = 240 units.
(1-1 correspondence
with each of the sets of 240 products.)
Each E8 lattice is
related to the 8-dimensional Hamming code.
The 480 octonion x-product orbits are
the two sets of 240 units.
John Baez, in a 2003-01-23 post to the sci.physics.research thread "The magic of 8", quoting his week 95 web page, said:
"... the lattice L ...[is] ... "even" ...[iff]... for any x in L the inner product x.x is even. This implies that L is integral, by the identity (x + y).(x + y) = x.x + 2x.y + y.y ... L ...[is]... "unimodular" ...[iff]... the volume of each lattice cell ... is 1. Another way to say it is this. Take any basis of L, that is, a bunch of vectors in L such that any vector in L can be uniquely expressed as an integer linear combination of these vectors. Then make a matrix with the components of these vectors as rows. Then take its determinant. That should equal plus or minus 1. Still another way to say it is this. We can define the "dual" of L, say L*, to be all the vectors x such that x.y is an integer for all y in L. An integer lattice is one that's contained in its dual, but L is unimodular if and only if L = L*. So people also call unimodular lattices "self-dual". ...... In dimension 8 there is only *one* even unimodular lattice (up to isometry), namely the wonderful lattice E8! The easiest way to think about this lattice is as follows. Say you are packing spheres in n dimensions in a checkerboard lattice --- in other words, you color the cubes of an n-dimensional checkerboard alternately red and black, and you put spheres centered at the center of every red cube, using the biggest spheres that will fit. There are some little hole left over where you could put smaller spheres if you wanted. And as you go up to higher dimensions, these little holes gets bigger! By the time you get up to dimension 8, there's enough room to put another sphere OF THE SAME SIZE AS THE REST in each hole! If you do that, you get the lattice E8. ...
... In dimension 16 there are only *two* even unimodular lattices. One is E8 + E8. A vector in this is just a pair of vectors in E8. The other is called D16+, which we get the same way as we got E8: we take a checkerboard lattice in 16 dimensions and stick in extra spheres in all the holes. More mathematically, to get E8 or D16+, we take all vectors in R^8 or R^16, respectively, whose coordinates are either *all* integers or *all* half-integers, for which the coordinates add up to an even integer. (A "half-integer" is an integer plus 1/2.) ...
[ The /\16 Barnes-Wall lattice (unimodular but odd)
has 2x240 + 16x240 = 480 + 3,840 = 4,320 units,
related to the 16-dimensional Reed-Muller code.
The 7,680 octonion xy-product orbits are
two sets of 3,840 units. ]
... in dimension 24, there are *24* even unimodular lattices, which were classified by Niemeier. A few of these are obvious, like E8 + E8 + E8 and E8 + D16+, but the coolest one is the "Leech lattice", which is the only one having no vectors of length 2. This is related to a whole WORLD of bizarre and perversely fascinating mathematics, like the "Monster group", the largest sporadic finite simple group --- and also to string theory. ... for now let me just describe how to get the Leech lattice. First of all, let's think about Lorentzian lattices, that is, lattices in Minkowski spacetime instead of Euclidean space. The difference is just that now the dot product is defined by(x_1,...,x_n) . (y_1,...,y_n) = - x_1 y_1 + x_2 y_2 + ... + x_n y_n
with the first coordinate representing time. It turns out that the only even unimodular Lorentzian lattices occur in dimensions of the form 8k + 2. There is only *one* in each of those dimensions, and it is very easy to describe: it consists of all vectors whose coordinates are either all integers or all half-integers, and whose coordinates add up to an even number. Note that the dimensions [are] of this form: 2, 10, 18, 26, etc., ... the 10-dimensional even unimodular Lorentzian lattice is pretty neat and has attracted some attention in string theory ... but the 26-dimensional one is even more neat. In particular, thanks to the cannonball trick of Lucas ...
... the number theorist Edouard Lucas in 1875 ... I assume this is the same Lucas who is famous for the Lucas numbers: 1,3,4,7,11,18,..., each one being the sum of the previous two, after starting off with 1 and 3. They are not quite as wonderful as the Fibonacci numbers, but in a study of pine cones it was found that while *most* cones have consecutive Fibonacci numbers of spirals going around clockwise and counterclockwise, a small minority of deviant cones use Lucas numbers instead. ... must have liked playing around with numbers, because in one publication he challenged his readers to prove that: "A square pyramid of cannon balls contains a square number of cannon balls only when it has 24 cannon balls along its base". In other words, the only integer solution of 1^2 + 2^2 + ... + n^2 = m^2, is the solution n = 24, not counting silly solutions like n = 0 and n = 1. It seems the Lucas didn't have a proof of this; the first proof is due to G. N. Watson in 1918, using hyperelliptic functions. ...... the vector v = (70,0,1,2,3,4,...,24) is "lightlike". In other words, v.v = 0 What this implies is that if we let T be the set of all integer multiples of v, and let S be the set of all vectors x in our lattice with x.v = 0, then T is contained in S, and S/T is a 24-dimensional lattice --- the Leech lattice! ... it means that if we do bosonic string theory in 26 dimensions on R^26 modulo the 26-dimensional even unimodular lattice, we get a theory having lots of symmetries related to those of the Leech lattice. In some sense this is a "maximally symmetric" approach to 26-dimensional bosonic string theory ...".
The /\24 Leech lattice has
3x240 + 3x16x240 + 3x16x16x240 =
= 720 + 11,520 + 184,320 = 196,560 units,
related to the 24-dimensional Golay code.
The 196,560 Leech lattice units,
plus 300 = symmetric part of 24x24,
plus 24
produce 196,884 which is the dimension
of a representation space of the Monster,
the largest sporadic finite simple group.
Richard E. Borcherds, in his paper What is Moonshine?, math.QA/9809110, describes
some of McKay's "... observations about the monster, which so far are completely unexplained. The monster has 9 conjugacy classes of elements that can be written as the product of two involutions of type 2A, and their orders are 1, 2, 3, 4, 5, 6, 2, 3, 4. McKay pointed out that these are exactly the numbers appearing on an affine E8 Dynkin diagram giving the linear relation between the simple roots. They are also the degrees of the irreducible representations of the binary icosahedral group. A similar thing happens for the baby monster: this time there are 5 classes of elements that are the product of two involutions of type 2A and their orders are 2, 4, 3, 2, 1. (This is connected with the fact that the baby monster is a 3,4-transposition group.) These are the numbers on an affine F4 Dynkin diagram, and if we take the double cover of an F4 Dynkin diagram we get an E7 Dynkin diagram. The number on an E7 Dynkin diagram are 1, 1, 2, 2, 3, 3, 4, 2 which are the dimensions of the irreducible representations of the binary octahedral group. The double cover of the baby monster is the centralizer of an element of order 2 in the monster. Finally a similar thing happens for Fi24.2 [where Fi24 is the Fischer group]: this time there are 3 classes of elements that are the product of two involutions of type 2A and their orders are 2, 3, 1. (This is connected with the fact that [Fi24.2] is a 3-transposition group.) These are the numbers on an affine G2 Dynkin diagram, and if we take the triple cover of an G2 Dynkin diagram we get an E6 Dynkin diagram. The number on an E6 Dynkin diagram are 1, 1, 1, 2, 2, 2, 3, which are the dimensions of the irreducible representations of the binary tetrahedral group. The triple cover of Fi24.2 is the centralizer of an element of order 3 in the monster. The connection between Dynkin diagrams and 3-dimensional rotation groups is well understood (and is called the McKay correspondence), but there is no known explanation for the connection with the monster. ..."
The octonion WXY-Cross-Product orbits should
also correspond to units.
The Euclidean lattices in 8, 16, and 24 dimensions
have Lorentz counterpart lattices in 10, 18, and 26 dimensions.
Lorentz spaces in k+2 dimensions are related to
the compact symmetric spaces Spin(6)/(Spin(4)xU(1),
Spin(10)/(Spin(8)xU(1)), E6/(Spin(10)xU(1)), and E7/(E6xU(1))
that occur in the A-D-E structure of the D4-D5-E6-E7 physics model.
The 26-dimensional Lorentz Leech lattice /\25,1 is
the lattice version of the J3(O)o traceless part
of the 27-dim Jordan algebra of Hermitian octonion matrices:
Re(O1) O4 O5
O4* Re(O2) O6
O5* O6* Re(O3)
Leech and Lorentz Leech lattices are described
in the book Sphere Packings, Lattices and Groups,
by Conway and Sloane, 3rd ed, Springer-Verlag 1999.
The Main Theorem of Chapter 27 states that
the group of all autochronous automorphisms of /\25,1
(autochronous = not interchanging past and future light cones)
is the split extension of the Coxeter group of /\25,1 whose
generators are the corresponding reflections
by the group Co(infinity) of all automorphisms of the Leech lattice
(including translations).
In /\25,1 the lightcone nullvectors include the vector
(0,1,2,3, ... ,21,22,23,24 | 70)
because the 24th square pyramidal number, 4900 = 70x70 = 70^2,
the sum of 0^2 + 1^2 + 2^2 + ... + 23^2 + 24^2,
is the only square pyramidal number that is itself a square.
The Leech roots of /\25,1 are those vectors r in /\25,1
that satisfy r.r = 2 and r.(0,1,2, ... ,23,24 | 70) = 0 .
The Leech roots in /\25,1 form a /\24 Leech lattice.
The Coxeter diagram for /\25,1 is actually infinite,
having one node for each Leech lattice vector.
Therefore:
THE LORENTZ LEECH LATTICE /\25,1 HAS FOR ITS COXETER DIAGRAM
THE LEECH LATTICE /\24
(so that the Lorentz Leech lattice, and therefore J3(O)o,
has a reflexive-recursive structure)
and
THE AUTOCHRONOUS AUTOMORPHISMS OF /\25,1
ACT TRANSITIVELY ON THE FUNDAMENTAL ROOTS OF /\25,1
In contrast, the Lorentz Barnes-Wall lattice /\17,1
has a finite Coxeter diagram:
x x
| |
x--x--x--x--x--x--x--x--x--x--x--x--x--x--x--x
with autochronous automorphism group of order 2,
and the Lorentz E8 lattice /\9,1
has a finite Coxeter diagram (denoted E10):
x
|
x--x--x--x--x--x--x--x--x
with trivial autochronous automorphism group.
3. SYMMETRY STRUCTURE OF A GIVEN OCTONION PRODUCT:
Onar Aam noticed that
any given product of octonions 1, i, j, k, E, I, J, K
contains within itself octonionic symmetry structure.
This section is based on the work of Onar Aam.
The 480 products are given (where S7 = permutations of 7) by
(Z2)^7 x S7 / (Z2)^3 x PSL(2,7)
Therefore each of the 480 products has
internal symmetry group (Z2)^3 x PSL(2,7)
so that PSL(2,7) is a symmetry group
of a given octonion product.
PSL(2,7) is double covered by 336-element SL(2,7).
SL(2,7) = Z2 x PSL(2,7) is also a symmetry group
of a given octonion product.
The 168-element simple group PSL(2,7) has
a 24-element octahedral (4,3,2) subgroup.
SL(2,7) has a 48-element binary octahedral {4,3,2} subgroup.
The binary octahedral {4,3,2} group is a subgroup of order 7
in SL(2,7).
For the given octonion product, there are
7 associative 3-dimensional quaternionic triangles:
i
/ \
k---j
i j k I J K
/ \ / \ / \ / \ / \ / \
K---J I---K J---I E---i j---E k---E
THEY CORRESPOND TO THE 7 IMAGINARY OCTONIONS,
and to the 7 coset spaces of SL(2,7) / {4,3,2}.
WHAT ABOUT THE REMAINING {4,3,2} INTERNAL SYMMETRY GROUP
within the SL(2,7), and
THE REMAINING (Z2)^2 INTERNAL SYMMETRY GROUP?
The binary octahedral {4,3,2} group can be represented
in quaternionic space as
the 24 vertices of a 24-cell
plus
the 24 vertices of the dual 24-cell.
Let the binary octahedral {4,3,2} group correspond to the
coassociative EIJK subspace of the
7-dimensional imaginary i, j, k, E, I, J, K octonion space.
EIJK has 4-dimensional quaternionic structure.
EIKJ
____
/ /\
/ / \
A=(34) / / \ B=(34)(23) counterclockwise
/ / /\ \ B=(23)(34) clockwise
/ / /\ \ \
/ /_/__\ \ \
/__________\ \ \
\ \ /
EIJK \_____________\/ EJIK
C=(23)
AA = BBB = CC = I = ABC if orientation is consistent.
The EIJK Escher triangle with permutations A, B, C represents
the 6-element dihedral (3,2,2) quotient group {4,3,2} / {2,2,2}
of the 48-element binary octahedral group
by the 8-element quaternion group.
The 8-element quaternion group corresponds to the
cyclic group Cy4 of order 4 (EIJK cycles)
times the cyclic group Z2 of order 2 (EIJK reversal reflections).
The 6 elements of the EIJK Escher triangle correspond
to 3 elements with clockwise orientation and
to 3 elements with counterclockwise orientation.
Recall we have also the internal symmetry group (Z2)^2.
THE TOTAL REMAINING INTERNAL SYMMETRY GROUP IS
Z2 x Z2 x Z2 x Cy4 x EIJK-Escher-6 of order 2x2x2x4x6 = 192.
It is the WEYL GROUP of Spin(0,8).
For more about these symmetries,
and octonion products,
and the Heptahedron and the Roman Steiner Surface,
see the corrected version of my April 1997 Corvallis talk.
Tony Smith's Home Page ......